
A capacitor
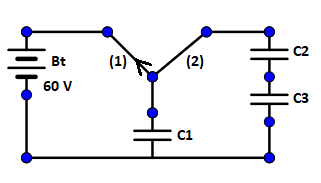
(A)
(B)
(C)
(D)

Answer
502.2k+ views
1 likes
Hint: The sum of the final charges an
Formulae Used:
Capacitor in series,
Where,
Potential difference in capacitor,
Where,
Complete step-by-step solution:
Given that,
A capacitor,
Voltage
The capacitance is,
Capacitor in series, and the capacitors
By rearranging the terms, then the above equation is written as,
By taking reciprocal on both sides of the above equation, then the above equation is written as,
By substituting the terms given terms in the above equation, then
On further simplification, then
On dividing, then the above equation is written as,
Now, the potential difference across three capacitors
By substituting the charge and the capacitance in the above equation, then
On further simplification, then
On dividing the terms in the above equation, then
The sum of the charge of the capacitors
Now, substituting the combined capacitance of
On multiplying, then
Thus, the above equation shows the sum of final charges
Hence, the option (A) is correct.
Note:- The capacitors
Formulae Used:
Capacitor in series,
Where,
Potential difference in capacitor,
Where,
Complete step-by-step solution:
Given that,
A capacitor,
Voltage
The capacitance is,
Capacitor in series, and the capacitors
By rearranging the terms, then the above equation is written as,
By taking reciprocal on both sides of the above equation, then the above equation is written as,
By substituting the terms given terms in the above equation, then
On further simplification, then
On dividing, then the above equation is written as,
Now, the potential difference across three capacitors
By substituting the charge and the capacitance in the above equation, then
On further simplification, then
On dividing the terms in the above equation, then
The sum of the charge of the capacitors
Now, substituting the combined capacitance of
On multiplying, then
Thus, the above equation shows the sum of final charges
Hence, the option (A) is correct.
Note:- The capacitors
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Derive an expression for electric potential at point class 12 physics CBSE

Who discovered the cell and how class 12 biology CBSE




