
How many 3 letter words can be formed using a, b, c, d, e if
A.repetition of letters is not allowed?
B.repetition of letters allowed?
Answer
530.1k+ views
Hint: In this question, we have to find the number of 3 letter words that can be formed using a, b, c, d, and e. If repetition is allowed then we can take that letter again. So, we have 5 intakes for every three places of 3 letter words.
Complete step-by-step answer:
The total number of words
Now, we have to find the number of 3 letter words if repetition is not allowed. That is we have to take 3 letters and we are given 5 letters. The number of 3 letter words that can be formed using 5 letters is
We know that words can be formed after rearranging also. So, the number of rearrangements is
Now, the total number of words that can be formed
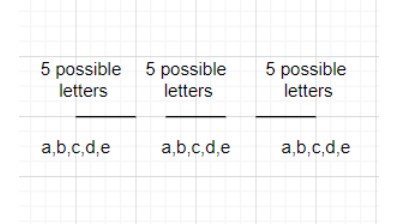
In case (ii), we have to find the number of three-letter words that can be formed if repetition is allowed. As repetition is allowed we can take that letter again if it is taken previously. In the first place, we can take a, b, c, d, and e. So, we have 5 intakes in the first place. Suppose, we take “a” in the first place of 3 letter words. We can also take the letter “a” in the second place too. So, in second place we can take a, b, c, d, and e. In the second place, we have 5 intakes. Similarly, in third place, we have 5 intakes.
The total number of words to be formed
Note: In this question, the mistake is generally done in finding the number of words that can be formed without repetition. As we have to take three letters out of five given letters, one can directly write

Complete step-by-step answer:
The total number of words
Now, we have to find the number of 3 letter words if repetition is not allowed. That is we have to take 3 letters and we are given 5 letters. The number of 3 letter words that can be formed using 5 letters is
We know that words can be formed after rearranging also. So, the number of rearrangements is
Now, the total number of words that can be formed
In case (ii), we have to find the number of three-letter words that can be formed if repetition is allowed. As repetition is allowed we can take that letter again if it is taken previously. In the first place, we can take a, b, c, d, and e. So, we have 5 intakes in the first place. Suppose, we take “a” in the first place of 3 letter words. We can also take the letter “a” in the second place too. So, in second place we can take a, b, c, d, and e. In the second place, we have 5 intakes. Similarly, in third place, we have 5 intakes.
The total number of words to be formed
Note: In this question, the mistake is generally done in finding the number of words that can be formed without repetition. As we have to take three letters out of five given letters, one can directly write
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE

What is the past tense of read class 10 english CBSE

In which type of rock coal is found A Igneous rock class 10 social science CBSE

What is the past participle of wear Is it worn or class 10 english CBSE




