




Factors of 11: An Introduction
Kids, do you ever find yourself struggling with prime numbers like 11,13, or 17 while arranging your bookshelf, dividing something into groups, rows, or columns, or even distributing sweets to your group of friends? Well, in such a case factors or factorization is all you need. A factor is a number that divides another number completely with no remainder. Factors and multiples are a part of our daily life. Factors are going to help you in various aspects of life. But when it comes to a prime number like 11 we often struggle to find its remainder, so, in this article, we will learn in detail about the Factors of 11, step-by-step calculation, Prime factor of 11, examples, and much more.
What is the Factor of a Number?
In maths, The factor of a number is a divisor that separates the number, without leaving any remainder in the end. To find the factor of a number, we can use various techniques like the division method and the multiplication technique.

Factor of 4.
The factors or of a numbeelements r can be positive or negative. Taking the number 8 as an example, let's find out what the variables are. Since 8 can be divided by 1, 2, 4, and 8, we can list the positive factors as 1, 2, 4, and 8. However, 8 has negative variables too, which can be written as, - 1, - 2, - 4, - 8 on the basis that the result of two negative numbers is a positive number, and that implies (- 1) × (- 8) = 8, and (- 2) × (- 4) = 8. However, we generally use just the positive factor of a number in our maths. Now let us move forward and learn some of the properties of a factor.
Analysing the Factor of 11
The number which completely divides 11 without leaving any remainder at the end is considered as the factor of 11.
Consequently, to get the factor of 11 we multiply the number into pairs which brings the original number 11. Since 11 is an indivisible number, it has just two factors: one and the actual number which is 11. In this manner, the factors of 11 are (1 and 11), and the negative elements of 11 are - 1 and - 11.
Factors of 11: 1 and 11.
Prime Factorization of 11: 1 × 11 or 11
Analysing the Factors of - 11?
To get the factor of -11 we multiply the two integers which bring the product to -11.
Example
-1 × 11 = - 11
1 × - 11 = - 11
By using (- 1, 11) as a couple of factors we can obtain -11., we can likewise use (1, - 11)as a couple of factors to obtain -11
How to Calculate the Factors of 11?
Step-by-step method of calculating factors of 11
We calculate the factors of 11 as per the following.
In the first place, we know that each number can be divided by 1 and itself without help from any other number
So, applying this logic, the factor of 11 is 1, and 11
By dividing various numbers by 1, 2, 3, and 4… we could find all factors of a number.
Step1: We know, 11 ÷ 1 = 11, it gives us the remainder as zero, and also it is divisible by itself. Thus we place it in our factors list
Now let's use the same process for other numbers.
1…. 11
Step 2: By dividing 11 ÷ 2 we get the remainder as 5.5. As here we do not get the remainder as zero, we cannot put this number in our factor list
Step 3: By dividing the number 11 by 3 we get the remaining portion as 3.66. Here, the remainder is not zero which means the number 11 is not entirely separated So we won't put this in our factor list either.
Step 4: By dividing the number 4 by 11 gives 11 ÷ 4 = 2.75, here, we are getting the remainder as 2.75 which is not getting divided. So we won't put four in our factors list
Step 5: Let's try this with our last number 5. 11 ÷ 5 = 2.2, Here, we are getting the remainder as 2.2 which is not getting entirely separated. So we won't put 5 in our factors list
Step 6: Since we have no more numbers to ascertain, we are putting the numbers up until this point.
So, through the above calculation, we conclude that 1 and 11 are the factors of 11.
Prime Factors of 11
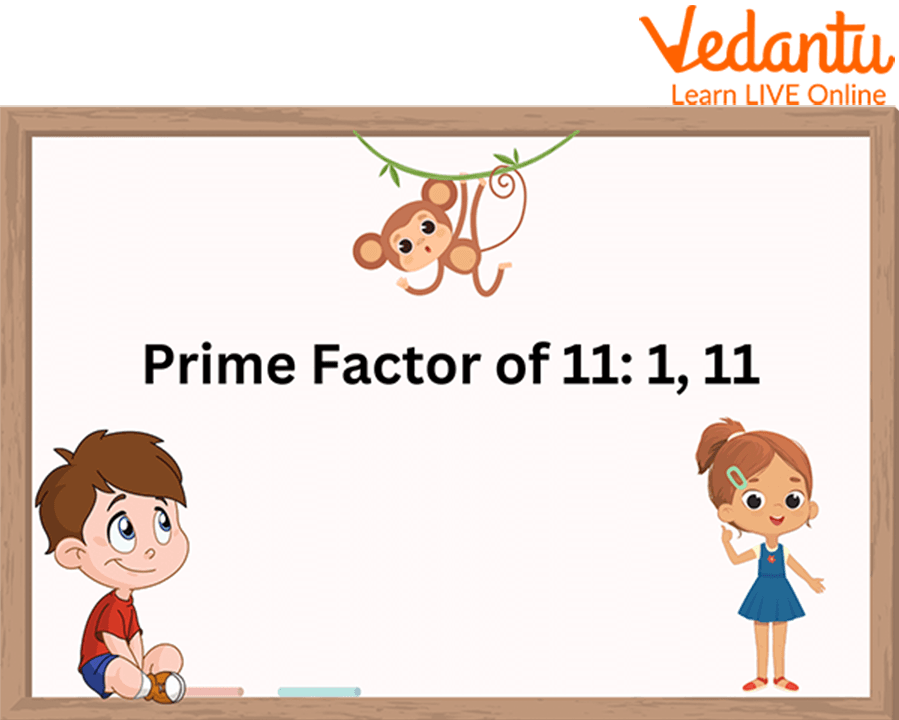
Prime Factor of 11
The prime factorization of a number is the process of expressing it as a product of prime numbers. The only prime numbers other than 1 and 11 that divide 11 exactly are 1 and 11, so by prime factorization, the prime factors of 11 are( 1 and 11.)
Since the only factors of 11 are 1 and 11, 11 is a prime number.
Finding Factors using Division Method
Using the division method, the factors of 11 are determined by dividing 11 by different integers. At the point when the integer numbers divide 11 precisely, then, at that point, those numbers are the Factors of 11.
Here are the factors of 11:
11/1 = 11 ( Factor is 1 and Remainder is 0 )
11/11 = 1 ( Factor is 11 and Remainder is 0 )
Solved Examples
Example 1: Determine the common factor of 8 and 11
Ans: The factors of 11 are 1 and 11.
The factors of 8 are 1,2,4 and 8
Here the common factor of the given numbers 8 and 10 is 1 as 11 is a prime number.
Example 2: Determine the common factor of 6 and 11
Ans: Factors of 11 = 1 and 11.
Factors of 6 = 1,2,3 and 6
The common factor of 11 and 6 is 1, as 11 is a prime number.
Example 3: Determine the common factor of 11 and 17
Ans:
The factors of 11 = 1 and 11.
The factors of 17 = 1 and 17
Here, both the numbers are prime numbers and the common factor of 11 and 17 is 1.
Practice Problems
Q 1. Find the common factors of the numbers 12 and 11.
Ans: Common Factor of 12 and 11 is 1.
Q 2. Find the common factor of the numbers 10 and 11.
Ans: Common Factor of 10 and 11 is 1.
Q 3. Find the common factor of the numbers 7 and 11.
Ans: Common Factor of 7 and 11 is 1.
Q 4. Find the common factor of the numbers 22 and 11.
Ans: Common Factor of 22 and 11 is 1 and 11.
Summary
In this article, we learned that the prime factor number ly ideas the given number until the final remainder is zero. Due to the indivisible nature of the number 11, we can only get its factor as 1 and 11. We also calculated step by step method into proving that 11 is not completely divided by any other number and we were only able to put the number 1 and 11 in our factors list. Through the prime factorization, we also got to know that the factors of 11 are 1 and 11 since they are the only factors and finally we used the division method to find the factors of 11.






FAQs on Factors of 11
1. Can 1 be a factor of all numbers?
Yes. Every number has a factor of 1 because when one divides a number exactly, leaving no remainder, the result is the number itself.
Eg:
1 is a factor of 7.
2. What is the real-world application of prime factorization?
In the real world, prime factorization is frequently used. Prime factorization is used, for instance, when we need to divide something into equal parts, when exchanging money, or when figuring out how long a journey will take. One typical illustration is when we need to divide a set of 21 chocolates among three children. We know the factors of 21 as 21 = 3 *7. This means that each child will get 7 candies.
3. How to use prime factorization to find LCM?
The Least Common Multiple is the abbreviation of LCM. The smallest number created when multiplying two or more numbers is known as the Least Common Multiple (LCM) of that number. Finding the prime factors of the two numbers will help you determine their LCM. The LCM is the result of the most powerful common prime factors. Let's determine the LCM of 12 and 11, for illustration. *The prime factorizations of 12 and 11 are respectively 2*2* 3*1 and 11*1. The combination of the factors with the highest powers among the common prime factors is 2*2 *3*11 = 132.
4. Why are there no prime factors for 0 and 1?
Number 1 represents the single factor and is neither prime nor composite as it only contains one factor. 0 can be equally divided through any number besides zero, hence it has an unlimited number of factors. 0 is therefore also regarded as neither prime nor composite.
5. Who is the inventor of prime numbers?
Greek mathematician Eratosthenes, devised an algorithm for calculating primes called the Sieve of Eratosthenes around 200 BC.
6. Which 4-digit number has the most factors?
The number which is found to be the greatest 4 digits is the number 9999 = 3 × 3 × 11 × 101 (A prime factor is the prime factor of a given number.) So the prime factors of the largest 4-digit number 9999 are 3, 3, 11, and 101.











