




Introduction to Addition
The process of combining two or more objects is known as an addition. The procedure of determining the sum of two or more integers in mathematics is known as an addition. The equation of the addition consists of the added along with the addition sign, and the result is called a sum. When we work with money, compute our shopping bills, or calculate time, we frequently employ addition.
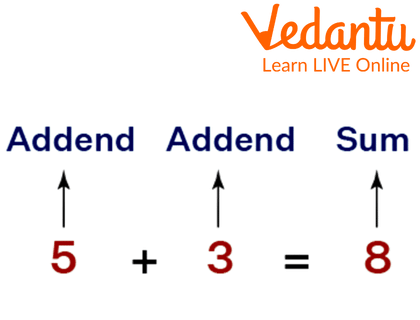
Formula for Addition
Definition of Like Terms and Unlike Terms
Like terms are ones that have the same variables and exponent power. The coefficients of these factors might vary. Algebraic expressions with like terms can be grouped together and then solved for addition.
Unlike terms are ones whose variables and exponents vary from one another. The algebraic equation has different variables and exponents like x, y, and z then the expression has, unlike terms. These unlike terms cannot be added together.
Like and Unlike Terms Examples
Let’s study the like and unlike terms with an example. Identify the like and unlike equations \[15x + 25y + 10\] and \[15x + 5x + 10\].
The condition for the like term is that only one variable should be present. so the equation with only one variable is \[15x + 5x + 10\] which can further be simplified or added to get \[20x + 10\]. But in the second equation, there are two variables x and y which makes the equation, unlike type. So, \[15x + 25y + 10\] is an example of an unlikely term.
If we were asked to add the terms \[15x + 25y + 10\] and \[20x + 10\] then we would have added the terms having the same variables. This means that we can add the coefficient of the variable x and the constant numbers. The coefficient with variable y would remain the same as the second equation does not contain the y variable part in it. So, if we add both the equation we will get the: \[\begin{array}{l}(15x + 25y + 10) + (20x + 10)\\35x + 25y + 20\end{array}\]
Conclusion
Operations like addition and subtraction need to be performed based on the like and unlike terms. Terms having the same variable can be added or subtracted. But the unlike terms can’t be added or subtracted due to different variables present. This method of addition makes it easier to calculate complex equations.
Sample Questions
1. \[3x + 3y\] is the
a. like term
b. unlike term
c. none of the above
d. can’t say
Ans: Unlike term
Explanation: They are unlike terms as two variables x and y are used in the equations and having the same coefficient does not mean that they are like terms.
2. \[3{x^3} + 14{x^2} + 10x\] is the
a. like term
b. unlike term
c. none of the above
d. can’t say
Ans: Unlike term
Explanation: The equation is unlike term and not like term as the variable x has different power present in it which makes it impossible to add together.
3. \[14x + 25x\] is the
a. like term
b. unlike term
c. none of the above
d. can’t say
Ans: Like term
Explanation: The equation is the like equation as only one variable (x) is present in the equation. The terms can be added together to get a final answer.
FAQs on Addition of Like and Unlike Terms
1. Can we combine like and unlike algebraic terms?
Ans: Any number of algebraic expressions can be added or subtracted. Unlike algebraic terms are those that do not have the same variables. It is not possible to add or delete terms from an expression.
2. What does it mean to combine like algebraic terms?
Ans: When an algebraic statement contains like terms or the same variables, that expression can be further reduced to the point where no additional computation is necessary. An expression can be quickly solved by merging comparable words. For example: \[2x + 14 - 56{x^2} + 20x\] can be simplified as \[22x + 14 - 56{x^2}\].
3. Can we combine, unlike algebraic terms?
Ans: No, unlike algebraic words, an algebraic expression cannot be merged or reduced further since it contains two distinct variables.
4. What is addition's identity property?
Ans: If 0 is added to any integer, the final total is always the real number, according to the identity characteristic of addition.
5. Where can we apply addition?
Ans: In daily life, we employ addition. For example, when we want to determine how much we used on the products we purchased, or the time it would take to complete a task, or the amount of materials needed in preparing anything, we must do the additional process.











