
The volume and curved surface area of the cylinder are 1650 cubic cm and 660 sq. cm respectively. Find the radius and height of the cylinder.
Answer
514.5k+ views
1 likes
Hint: Let us assume the radius and height of the cylinder as ‘r’ and h respectively. We have given the volume and curved surface area of the cylinder. We know that the formula for the volume of the cylinder is
Complete step-by-step solution:
We have given the volume and curved surface area of the cylinder as 1650 cubic cm and 660 sq. cm respectively.
Let us assume that the radius and height of the cylinder are r and h respectively.
In the below diagram, we have shown a cylinder with radius (r) and height (h).
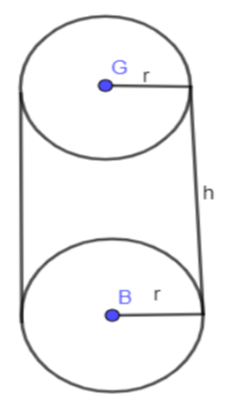
We know the formula for volume of the cylinder is equal to:
Equating the above volume to 1650 we get,
We know the formula for curved surface area of the cylinder is:
Equating the above curved surface area to 660 we get,
Now, we have two equations and two unknowns r and h which we are going to solve by dividing eq. (1) by eq. (2).
In the above equation,
Multiplying 2 on both the sides we get
Substituting the above value of r in eq. (2) we get,
Substituting the value of
Cross multiplying the above equation we get,
Hence, we have got the value of radius (r) as 5 cm and height (h) as 21 cm.
Note: You can verify that the value of the radius and height of the cylinder that you solved above is correct or not by substituting these values in the formula of the curved surface area of the cylinder.
The formula of curved surface area for cylinder is equal to:
Substituting the value of r and h as 5 cm and 21 cm respectively in the above equation we get,
21 will be cancelled out 3 times by 7 in the above expression and we get,
Now, as you can see in the question given above, the curved surface area of the cylinder is
Hence, the value of the radius and height of the cylinder that we are getting is correct.
Complete step-by-step solution:
We have given the volume and curved surface area of the cylinder as 1650 cubic cm and 660 sq. cm respectively.
Let us assume that the radius and height of the cylinder are r and h respectively.
In the below diagram, we have shown a cylinder with radius (r) and height (h).

We know the formula for volume of the cylinder is equal to:
Equating the above volume to 1650 we get,
We know the formula for curved surface area of the cylinder is:
Equating the above curved surface area to 660 we get,
Now, we have two equations and two unknowns r and h which we are going to solve by dividing eq. (1) by eq. (2).
In the above equation,
Multiplying 2 on both the sides we get
Substituting the above value of r in eq. (2) we get,
Substituting the value of
Cross multiplying the above equation we get,
Hence, we have got the value of radius (r) as 5 cm and height (h) as 21 cm.
Note: You can verify that the value of the radius and height of the cylinder that you solved above is correct or not by substituting these values in the formula of the curved surface area of the cylinder.
The formula of curved surface area for cylinder is equal to:
Substituting the value of r and h as 5 cm and 21 cm respectively in the above equation we get,
21 will be cancelled out 3 times by 7 in the above expression and we get,
Now, as you can see in the question given above, the curved surface area of the cylinder is
Hence, the value of the radius and height of the cylinder that we are getting is correct.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




