
The sum of the slopes of the lines tangent to both the circles
Answer
518.4k+ views
Hint: To find the slope of the line joining two points
Complete step-by-step answer:
As we know that the standard equation of the circle is
So, comparing equations of both of the circles to get their radius and centre.
So, the centre of the circle
And the centre of the circle
Now let us plot both the circles in the coordinate plane.
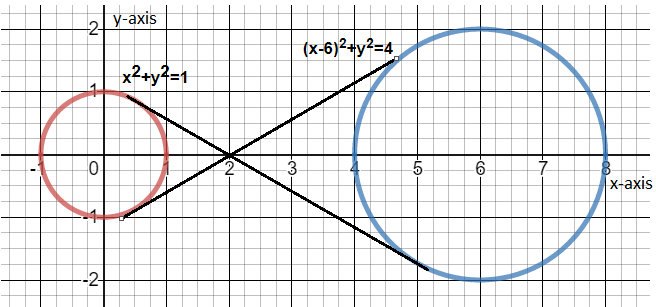
Now we can see from the above figure that both the circles are symmetric around the x-axis.
So, the angle made by the common tangents to both of the circles will be equal and opposite.
And as we know that the tan of the angle made any line with x axis is the slope of the line.
So, the slopes of the common tangents to both the circles will be equal and opposite.
Hence, the sum of the slopes of the tangents to the circle
Note: Whenever we come up with this type of problem we should first, first find the centre and the radius of the given circles by comparing their equation with the standard equation of circle that is
Complete step-by-step answer:
As we know that the standard equation of the circle is
So, comparing equations of both of the circles to get their radius and centre.
So, the centre of the circle
And the centre of the circle
Now let us plot both the circles in the coordinate plane.

Now we can see from the above figure that both the circles are symmetric around the x-axis.
So, the angle made by the common tangents to both of the circles will be equal and opposite.
And as we know that the tan of the angle made any line with x axis is the slope of the line.
So, the slopes of the common tangents to both the circles will be equal and opposite.
Hence, the sum of the slopes of the tangents to the circle
Note: Whenever we come up with this type of problem we should first, first find the centre and the radius of the given circles by comparing their equation with the standard equation of circle that is
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




