
The perimeter of an isosceles triangle is 42 cm and its base is
Answer
493.2k+ views
Hint: In this question, we need to determine the area of the isosceles triangle such that the perimeter of an isosceles triangle is 42 cm and its base is
Complete step-by-step answer:
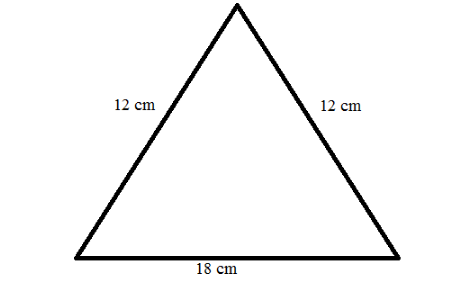
An isosceles triangle is the one which has two equal sides and the length of the base is different from the length of the equal sides. The equal sides often known as congruent sides.
Let the length of the congruent sides of the isosceles triangle be
According to the question, the length of the base of the isosceles triangle is
The length of the base is given as,
The sum of all the sides of the triangle is the perimeter of the triangle.
Also, it has been given that the perimeter of the isosceles triangle is 42 cm. So, we can write
Hence, the length of the sides of the isosceles triangle are 12 cm, 12 cm and
Now, using Heron's formula to evaluate the area of the triangle as
So,
Substituting the value of ‘s’ in the formula
Hence, the area of the isosceles triangle is 71.435 sq. cm. which approximates to 72 sq.cm.
So, the correct answer is “72.sq. cm”.
Note: It is worth noting down here that the Heron's formula can only be used to find the area of the triangle of which the length of each side has been given. However, if the length of the base and the length of the triangles are known then, we can use the formula
Complete step-by-step answer:

An isosceles triangle is the one which has two equal sides and the length of the base is different from the length of the equal sides. The equal sides often known as congruent sides.
Let the length of the congruent sides of the isosceles triangle be
According to the question, the length of the base of the isosceles triangle is
The length of the base is given as,
The sum of all the sides of the triangle is the perimeter of the triangle.
Also, it has been given that the perimeter of the isosceles triangle is 42 cm. So, we can write
Hence, the length of the sides of the isosceles triangle are 12 cm, 12 cm and
Now, using Heron's formula to evaluate the area of the triangle as
So,
Substituting the value of ‘s’ in the formula
Hence, the area of the isosceles triangle is 71.435 sq. cm. which approximates to 72 sq.cm.
So, the correct answer is “72.sq. cm”.
Note: It is worth noting down here that the Heron's formula can only be used to find the area of the triangle of which the length of each side has been given. However, if the length of the base and the length of the triangles are known then, we can use the formula
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
The Chinese traveller FaHien visited India and left class 8 social science CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

How many ounces are in 500 mL class 8 maths CBSE

Name the states through which the Tropic of Cancer class 8 social science CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Is the past tense for sink sank or sunk class 8 english CBSE




