
The number of 5 digit telephone numbers having least one of their digits repeated.
a) 90,000
b) 100,000
c) 30,240
d) 69,760
Answer
537.3k+ views
Hint: At least one digit repeated numbers can be given by the difference of total numbers of 5 digits from the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9. with allowed repetition and the numbers with no repetition (distinct digits). Selection of ‘r’ different things from the total ‘n’ different things is given by
Complete step-by-step answer:
As we know there are 10 digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 that can be used for making a 5 digit telephone number and as we need to determine total telephonic numbers of 5 digits having at least one digit repeated. It means there may be repetition of one digit or repetition of two digits or three but all the digits involved for making 5 digits cannot be distinct to each other i.e. at least one digit should be repeated.
So, in other words we need to calculate all the 5 digit numbers except the numbers which have all 5 digit different. It means total telephonic numbers with 5 digits having at least one digit repeated can be calculated by the difference of total numbers of 5 digits and the numbers with all distinct 5 digits. So, total 5 digit numbers with the help of 10 digit numbers and repetition is allowed with the formation of numbers can be given as
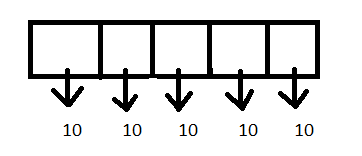
As digits can be repeated it means there are 10 possibilities for a single digit. Hence, total 5 digit numbers can be given as
Now, as we know, selection of r different things from ‘n’ different numbers is given by relation
Now, as we know that the total 5 digit numbers with no repetition can be calculated as
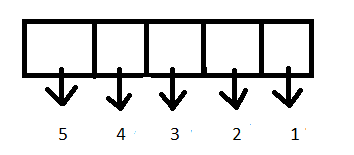
The first digit can be filled by 5 ways and second by 4 because the same digit cannot be occupied at two places as repetition is not allowed. Similarly, 3rd place can be filled by 3 ways as two digits are already occupied with two different numbers. Hence, we can get total number of 5 digits telephonic number with 5 selected digits
So, numbers with at least one digit repeated are given by the difference of total numbers possible with repetition and without repetition. So, we get total 5 digit telephone numbers with having at least one of the digit repeated
= 100,000 – 30240
= 69760.
Hence, option (d) is correct.
Note: One may go wrong if he or she select 5 digits from the given numbers by
One may arrange the 5 different digits by 5! Ways directly for the second case in the solution. The arrangement of ‘n’ different things can be given by formula n!.
Complete step-by-step answer:
As we know there are 10 digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 that can be used for making a 5 digit telephone number and as we need to determine total telephonic numbers of 5 digits having at least one digit repeated. It means there may be repetition of one digit or repetition of two digits or three but all the digits involved for making 5 digits cannot be distinct to each other i.e. at least one digit should be repeated.
So, in other words we need to calculate all the 5 digit numbers except the numbers which have all 5 digit different. It means total telephonic numbers with 5 digits having at least one digit repeated can be calculated by the difference of total numbers of 5 digits and the numbers with all distinct 5 digits. So, total 5 digit numbers with the help of 10 digit numbers and repetition is allowed with the formation of numbers can be given as

As digits can be repeated it means there are 10 possibilities for a single digit. Hence, total 5 digit numbers can be given as
Now, as we know, selection of r different things from ‘n’ different numbers is given by relation
Now, as we know that the total 5 digit numbers with no repetition can be calculated as

The first digit can be filled by 5 ways and second by 4 because the same digit cannot be occupied at two places as repetition is not allowed. Similarly, 3rd place can be filled by 3 ways as two digits are already occupied with two different numbers. Hence, we can get total number of 5 digits telephonic number with 5 selected digits
So, numbers with at least one digit repeated are given by the difference of total numbers possible with repetition and without repetition. So, we get total 5 digit telephone numbers with having at least one of the digit repeated
= 100,000 – 30240
= 69760.
Hence, option (d) is correct.
Note: One may go wrong if he or she select 5 digits from the given numbers by
One may arrange the 5 different digits by 5! Ways directly for the second case in the solution. The arrangement of ‘n’ different things can be given by formula n!.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




