
The length of the diagonals of a rhombus is 24cm and 18cm. Find the length of each side of the rhombus.
Answer
536.1k+ views
Hint:Calculate the half-length of both the diagonals of the rhombus. Use the fact that the diagonals of a rhombus bisect each other and then use Pythagoras Theorem to calculate the length of each side of the rhombus.
Complete step-by-step answer:
We have to calculate the length of each side of a rhombus whose diagonals are of length 18cm and 24cm.
We will consider the rhombus ABCD whose diagonals intersect at point O such that
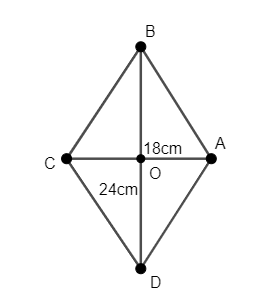
We will first calculate the half-length of both the diagonals of the rhombus.
We know that the diagonals of a rhombus bisect each other.
Thus, we have
As the diagonals bisect each other, we have
We will now use Pythagoras Theorem to calculate the length of each side of the rhombus.
We know that Pythagoras Theorem states that in a right-angled triangle, the sum of squares of two perpendicular sides is equal to the square of the third side.
Thus, in
Substituting
Thus, we have
Taking square root on both sides, we have
We know that the length of all sides of a rhombus is equal. Thus, we have
Hence, the length of each side of the rhombus is 15cm.
Note: We can calculate the length of each side of rhombus by applying Pythagoras Theorem in any of the right-angled triangles. We will get the same answer in each case. We don’t have to calculate the length of each side of the rhombus. We can simply use the fact that the length of all sides of a rhombus is equal.Remember that the length of diagonals of rhombus are always different and they only bisect with each other.
Complete step-by-step answer:
We have to calculate the length of each side of a rhombus whose diagonals are of length 18cm and 24cm.
We will consider the rhombus ABCD whose diagonals intersect at point O such that

We will first calculate the half-length of both the diagonals of the rhombus.
We know that the diagonals of a rhombus bisect each other.
Thus, we have
As the diagonals bisect each other, we have
We will now use Pythagoras Theorem to calculate the length of each side of the rhombus.
We know that Pythagoras Theorem states that in a right-angled triangle, the sum of squares of two perpendicular sides is equal to the square of the third side.
Thus, in
Substituting
Thus, we have
Taking square root on both sides, we have
We know that the length of all sides of a rhombus is equal. Thus, we have
Hence, the length of each side of the rhombus is 15cm.
Note: We can calculate the length of each side of rhombus by applying Pythagoras Theorem in any of the right-angled triangles. We will get the same answer in each case. We don’t have to calculate the length of each side of the rhombus. We can simply use the fact that the length of all sides of a rhombus is equal.Remember that the length of diagonals of rhombus are always different and they only bisect with each other.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

When and how did Canada eventually gain its independence class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




