Answer
282.6k+ views
Hint: In the given question, we are required to find the equations of the tangents to the ellipse whose equation is provided to us in the question from the point $\left( {2,3} \right)$. So, we first find the general equation of the line passing through the point $\left( {2,3} \right)$ and then apply the condition of tangency with the ellipse to get to the required answer.
Complete answer:
Firstly, we have the point $\left( {2,3} \right)$. So, we find the equation of the line passing through this point.
We know the slope intercept form as $y = mx + c$. So, we substitute x as $2$ and y as $3$.
Hence, we get,
$ \Rightarrow 3 = 2m + c$
Finding value of c in terms of m, we get,
$ \Rightarrow c = 3 - 2m$
So, we have the equation line passing through $\left( {2,3} \right)$ as $y = mx + \left( {3 - 2m} \right) - - - - - \left( 1 \right)$.
Now, we have the equation of the ellipse as $9{x^2} + 16{y^2} = 144$.
Dividing both sides by $144$, we have,
\[ \Rightarrow \dfrac{{{x^2}}}{{16}} + \dfrac{{{y^2}}}{9} = 1\]
Now, we can see that the equation of the ellipse resembles with \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\], where a is greater than b.
We get ${a^2} = 16$ and ${b^2} = 9$
So, the value of a is $4$ and that of b is $3$.
Now, we know the condition of tangency of a line $y = mx + c$ with an ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] is ${c^2} = {a^2}{m^2} + {b^2}$.
So, we get, ${\left( {3 - 2m} \right)^2} = 16{m^2} + 9$
Evaluating the whole square using algebraic identity ${\left( {a - b} \right)^2} = {a^2} - 2ab + {b^2}$, we get,
$ \Rightarrow 9 + 4{m^2} - 12m = 16{m^2} + 9$
Adding up like terms and solving for m, we get,
$ \Rightarrow 12{m^2} + 12m = 0$
$ \Rightarrow 12m\left( {m + 1} \right) = 0$
So, either $m = 0$ or $\left( {m + 1} \right) = 0$
$ \Rightarrow m = 0$ or $ \Rightarrow m = - 1$
So, the value of m is zero or $ - 1$.
Substituting the value of m as zero in equation $\left( 1 \right)$, we get,
$y = 0x + \left( {3 - 2 \times 0} \right)$
Simplifying calculations, we get,
$y = 3$
Now, substituting m as $ - 1$, we get,
$y = \left( { - 1} \right)x + \left( {3 - 2 \times \left( { - 1} \right)} \right)$
$ \Rightarrow y + x = 5$
So, the equations of the tangents to the ellipse $9{x^2} + 16{y^2} = 144$ from the point $\left( {2,3} \right)$ are: $y + x = 5$ and $y = 3$.
Hence, option (D) is the correct answer.
Note:
The values of a and b in the equation of the ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] represent the lengths of semi-major and semi-minor axes of an ellipse and hence the values cannot be negative. The condition of tangency for a line with ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] is ${c^2} = {a^2}{m^2} + {b^2}$ where the equation of the line is $y = mx + c$.
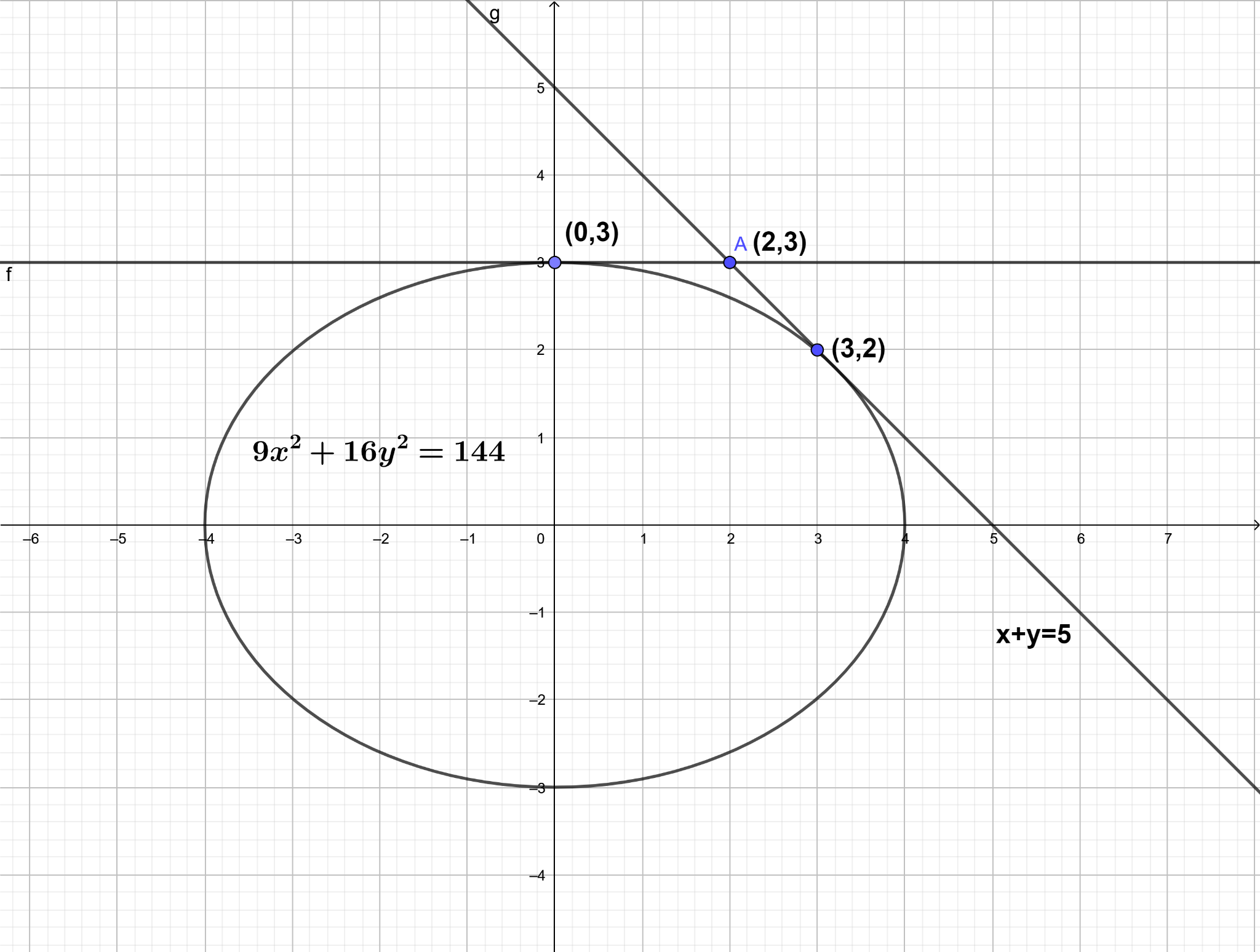
Complete answer:
Firstly, we have the point $\left( {2,3} \right)$. So, we find the equation of the line passing through this point.
We know the slope intercept form as $y = mx + c$. So, we substitute x as $2$ and y as $3$.
Hence, we get,
$ \Rightarrow 3 = 2m + c$
Finding value of c in terms of m, we get,
$ \Rightarrow c = 3 - 2m$
So, we have the equation line passing through $\left( {2,3} \right)$ as $y = mx + \left( {3 - 2m} \right) - - - - - \left( 1 \right)$.
Now, we have the equation of the ellipse as $9{x^2} + 16{y^2} = 144$.
Dividing both sides by $144$, we have,
\[ \Rightarrow \dfrac{{{x^2}}}{{16}} + \dfrac{{{y^2}}}{9} = 1\]
Now, we can see that the equation of the ellipse resembles with \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\], where a is greater than b.
We get ${a^2} = 16$ and ${b^2} = 9$
So, the value of a is $4$ and that of b is $3$.
Now, we know the condition of tangency of a line $y = mx + c$ with an ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] is ${c^2} = {a^2}{m^2} + {b^2}$.
So, we get, ${\left( {3 - 2m} \right)^2} = 16{m^2} + 9$
Evaluating the whole square using algebraic identity ${\left( {a - b} \right)^2} = {a^2} - 2ab + {b^2}$, we get,
$ \Rightarrow 9 + 4{m^2} - 12m = 16{m^2} + 9$
Adding up like terms and solving for m, we get,
$ \Rightarrow 12{m^2} + 12m = 0$
$ \Rightarrow 12m\left( {m + 1} \right) = 0$
So, either $m = 0$ or $\left( {m + 1} \right) = 0$
$ \Rightarrow m = 0$ or $ \Rightarrow m = - 1$
So, the value of m is zero or $ - 1$.
Substituting the value of m as zero in equation $\left( 1 \right)$, we get,
$y = 0x + \left( {3 - 2 \times 0} \right)$
Simplifying calculations, we get,
$y = 3$
Now, substituting m as $ - 1$, we get,
$y = \left( { - 1} \right)x + \left( {3 - 2 \times \left( { - 1} \right)} \right)$
$ \Rightarrow y + x = 5$
So, the equations of the tangents to the ellipse $9{x^2} + 16{y^2} = 144$ from the point $\left( {2,3} \right)$ are: $y + x = 5$ and $y = 3$.
Hence, option (D) is the correct answer.
Note:
The values of a and b in the equation of the ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] represent the lengths of semi-major and semi-minor axes of an ellipse and hence the values cannot be negative. The condition of tangency for a line with ellipse \[\dfrac{{{x^2}}}{a} + \dfrac{{{y^2}}}{b} = 1\] is ${c^2} = {a^2}{m^2} + {b^2}$ where the equation of the line is $y = mx + c$.

Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Assertion The resistivity of a semiconductor increases class 13 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the stopping potential when the metal with class 12 physics JEE_Main

The momentum of a photon is 2 times 10 16gm cmsec Its class 12 physics JEE_Main

Using the following information to help you answer class 12 chemistry CBSE

Trending doubts
Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Write an application to the principal requesting five class 10 english CBSE

Difference Between Plant Cell and Animal Cell

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What organs are located on the left side of your body class 11 biology CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE



