
The diagonals of a rhombus are 10cm and 24cm. Find the length of each side of a rhombus.
Answer
498.6k+ views
1 likes
Hint: Let us draw a rough figure as follows
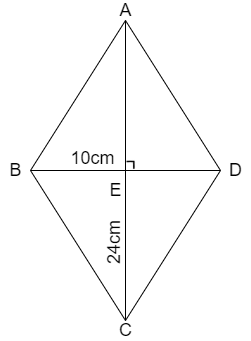
We solve this problem by using the definition of rhombus that is all the sides of rhombus are equal. We also use the condition that the diagonals intersect at right angles at mid – points. For finding the side length we use the Pythagoras Theorem states that the square of hypotenuse is equal to sum of squares of other two sides that is for the triangle shown below

The Pythagoras theorem is given as
Complete step by step answer:
We are given that the length of diagonals as 10cm and 24cm
Let us assume that from the figure the length of diagonals as
We know that the diagonals of rhombus intersect at right angles at mid – points that means the point ‘E’ is mid – point of both ‘AC’ and ‘BD’
By using the above condition the length of ED can be calculated as
By substituting the required values we get
Similarly, by using the condition that point ‘E’ is mid – point of ‘AC’ we get
By substituting the required values we get
Now, let us consider the triangle
We know that the Pythagoras Theorem states that the square of hypotenuse is equal to sum of squares of other two sides that is for the triangle shown below
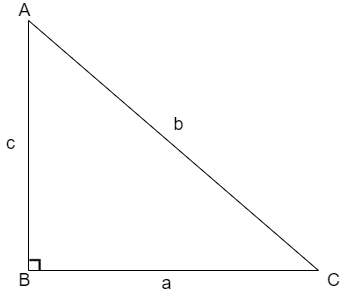
The Pythagoras theorem is given as
By using the Pythagoras theorem to triangle
By substituting the required values in above equation we get
We know that all the sides of a rhombus are equal
By using the above condition we get
Therefore the length of each side of a given rhombus is 13cm.
Note: We can solve this problem in another method.
If
If
Since the area will be same in any method by equating them we get
Now by squaring on both sides we get
Now, by substituting the required values we get
Therefore the length of each side of a given rhombus is 13cm.

We solve this problem by using the definition of rhombus that is all the sides of rhombus are equal. We also use the condition that the diagonals intersect at right angles at mid – points. For finding the side length we use the Pythagoras Theorem states that the square of hypotenuse is equal to sum of squares of other two sides that is for the triangle shown below

The Pythagoras theorem is given as
Complete step by step answer:
We are given that the length of diagonals as 10cm and 24cm
Let us assume that from the figure the length of diagonals as
We know that the diagonals of rhombus intersect at right angles at mid – points that means the point ‘E’ is mid – point of both ‘AC’ and ‘BD’
By using the above condition the length of ED can be calculated as
By substituting the required values we get
Similarly, by using the condition that point ‘E’ is mid – point of ‘AC’ we get
By substituting the required values we get
Now, let us consider the triangle
We know that the Pythagoras Theorem states that the square of hypotenuse is equal to sum of squares of other two sides that is for the triangle shown below

The Pythagoras theorem is given as
By using the Pythagoras theorem to triangle
By substituting the required values in above equation we get
We know that all the sides of a rhombus are equal
By using the above condition we get
Therefore the length of each side of a given rhombus is 13cm.
Note: We can solve this problem in another method.
If
If
Since the area will be same in any method by equating them we get
Now by squaring on both sides we get
Now, by substituting the required values we get
Therefore the length of each side of a given rhombus is 13cm.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What is the past tense of read class 10 english CBSE

In which type of rock coal is found A Igneous rock class 10 social science CBSE

Which one of the following is the deepest seaport of class 10 social science CBSE




