
The curved surface area of a cone of slant height
A.
B.
C.
D.
Answer
487.5k+ views
Hint: We know that, the curved surface area
At first, we will find the arc length of the sector, the circumference of the circle and the area of the circle.
Using the above formula and information we get the curved surface area.
Complete step-by-step solution:
Cone with slant height
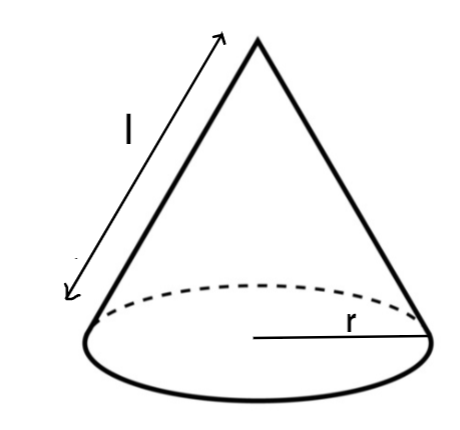
It is given that; the slant height of a cone is
We have to find the curved surface area of the cone.
We know that, the curved surface area
If a perpendicular cut is made from a point on the circumference of the base to the vertex and the cone is opened up, a sector of a circle with radius
Now, substitute these values in the above given formula we get,
The curved surface area
Simplifying we get,
The curved surface area
Hence, the correct option is B.
Note: A cone is a shape formed by using a set of line segments or the lines which connects a common point, called the apex or vertex, to all the points of a circular base
The curved surface area is defined as the area of only curved surfaces, leaving the circular top and base.
The curved surface area of a right circular cone equals the perimeter of the base times one-half slant height.
At first, we will find the arc length of the sector, the circumference of the circle and the area of the circle.
Using the above formula and information we get the curved surface area.
Complete step-by-step solution:
Cone with slant height

It is given that; the slant height of a cone is
We have to find the curved surface area of the cone.
We know that, the curved surface area
If a perpendicular cut is made from a point on the circumference of the base to the vertex and the cone is opened up, a sector of a circle with radius
Now, substitute these values in the above given formula we get,
The curved surface area
Simplifying we get,
The curved surface area
Hence, the correct option is B.
Note: A cone is a shape formed by using a set of line segments or the lines which connects a common point, called the apex or vertex, to all the points of a circular base
The curved surface area is defined as the area of only curved surfaces, leaving the circular top and base.
The curved surface area of a right circular cone equals the perimeter of the base times one-half slant height.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




