
State true or false:
Angles forming a linear pair are supplementary.
A. True
B. False
Answer
495k+ views
2 likes
Hint: In this question, we need to check if angles forming a linear pair are supplementary or not. For this, we need to prove if angles forming a linear pair form a sum of
Complete step-by-step solution
Linear pairs of angles are formed when two lines intersect each other at a single point. The angles are said to be linear if they are adjacent to each other after the intersection of the two lines. Now we need to prove that angles forming a linear pair are supplementary, that is, the sum of angles forming a linear pair is
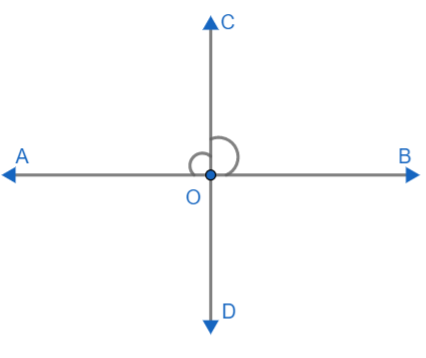
Now, as we can see
From the diagram, we can say that, the sum of
Hence,
Since,
Now we get,
As we can see, the sum of
Hence, the statement is true.
Therefore, option A is the correct answer.
Note: For solving this sum, students should know the meaning of the term linear pair and supplementary angles. Students can get confused between the terms supplementary angle and complementary angle. The angle whose sum is
Complete step-by-step solution
Linear pairs of angles are formed when two lines intersect each other at a single point. The angles are said to be linear if they are adjacent to each other after the intersection of the two lines. Now we need to prove that angles forming a linear pair are supplementary, that is, the sum of angles forming a linear pair is

Now, as we can see
From the diagram, we can say that, the sum of
Hence,
Since,
Now we get,
As we can see, the sum of
Hence, the statement is true.
Therefore, option A is the correct answer.
Note: For solving this sum, students should know the meaning of the term linear pair and supplementary angles. Students can get confused between the terms supplementary angle and complementary angle. The angle whose sum is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

One lakh eight thousand how can we write it in num class 7 maths CBSE

What is meant by Indian Standard Time Why do we need class 7 social science CBSE





