
How do you solve the equation
Answer
477.9k+ views
Hint: Here, we will first find the
Complete step-by-step answer:
The given quadratic equation is:
Now, in order to plot a graph of this equation, we will, first of all, determine the
Hence, we will substitute
Hence,
Thus, the
Now, we will determine the
Doing middle term split, we get
Factoring out common terms, we get
By using zero product property, we get
Or
Therefore, for
Now, we will determine the vertex by using the formula
In the given equation,
Thus, substituting these values in
Substituting this is the equation
Simplifying the expression, we get
Therefore, the vertex is:
Also, let us substitute
Simplifying the expression, we get
Therefore, the other point is
Hence, we will plot the graph having:
Vertex:
Extra point:
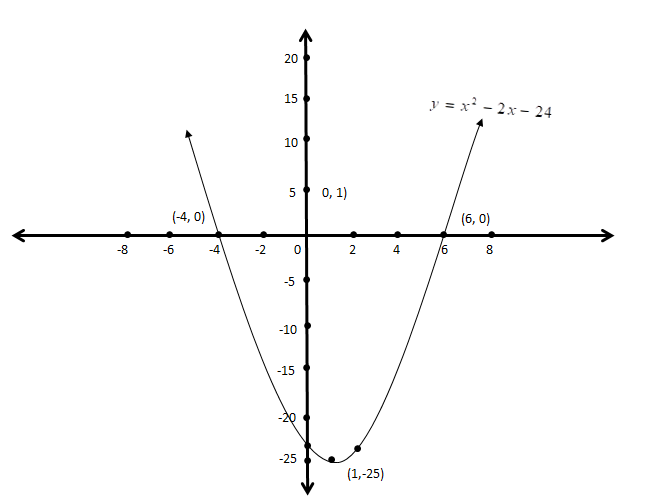
Hence, from this graph, we can clearly observe that the values
Therefore, this is the required solution of the given equation
Hence, this is the required answer.
Note:
An equation is called a quadratic equation if it can be written in the form of
Complete step-by-step answer:
The given quadratic equation is:
Now, in order to plot a graph of this equation, we will, first of all, determine the
Hence, we will substitute
Hence,
Thus, the
Now, we will determine the
Doing middle term split, we get
Factoring out common terms, we get
By using zero product property, we get
Or
Therefore, for
Now, we will determine the vertex by using the formula
In the given equation,
Thus, substituting these values in
Substituting this is the equation
Simplifying the expression, we get
Therefore, the vertex is:
Also, let us substitute
Simplifying the expression, we get
Therefore, the other point is
Hence, we will plot the graph having:
Vertex:
Extra point:

Hence, from this graph, we can clearly observe that the values
Therefore, this is the required solution of the given equation
Hence, this is the required answer.
Note:
An equation is called a quadratic equation if it can be written in the form of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




