
How do I solve for a right triangle?
Answer
460.5k+ views
Hint: We figure out the underlying concept behind the problem. This particular problem is under the topic of the solution of triangles and to some extent of trigonometry. For solving this problem first of all we need to understand the given problem statement clearly. To solve for a right triangle, we need to find the values of all the sides of the triangle as well as all the angles of the triangle. What we can do is find the ratio of the sides and then assume that the length of one of the sides is
Complete step by step solution:
Now we start off with the solution to the given problem by writing that the two sides of the right triangle are equal which also refers that their opposite angles are also equal. One of the sides of a right triangle is
Now if we assume the length of the base of the given triangle is
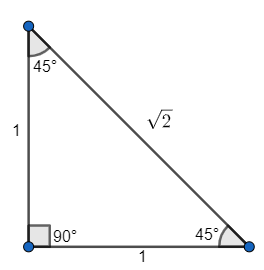
Note: These kinds of problems require an in-depth understanding of the different properties of triangles as well as to some extent trigonometry. This particular problem can also be solved using pure trigonometry by applying sine, cosine or tangent to any of the angles to find the required side length. This method however is a bit lengthy as we need to dive a little bit into trigonometry, but it is quite easy to remember and apply. One important thing to remember is that we have to assume one of the sides of the triangle and the rest can be found out accordingly.
Complete step by step solution:
Now we start off with the solution to the given problem by writing that the two sides of the right triangle are equal which also refers that their opposite angles are also equal. One of the sides of a right triangle is
Now if we assume the length of the base of the given triangle is

Note: These kinds of problems require an in-depth understanding of the different properties of triangles as well as to some extent trigonometry. This particular problem can also be solved using pure trigonometry by applying sine, cosine or tangent to any of the angles to find the required side length. This method however is a bit lengthy as we need to dive a little bit into trigonometry, but it is quite easy to remember and apply. One important thing to remember is that we have to assume one of the sides of the triangle and the rest can be found out accordingly.
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




