
How many sides does regular polygon have, whose interior angle is eight times its exterior angle?
(a)16
(b)24
(c)18
(d)20
Answer
521.7k+ views
2 likes
Hint: First find a formula for an interior angle in an n-sided polygon. Now you get a relation between angle and number of sides. So, by using the first condition you can derive an equation in terms of the number of sides of both the polygons. By using the second condition. Now you have 2 equations in the same variables, so by applying elimination method you can equate the both equations. Thus, getting the value of the number of sides.
Complete step-by-step answer:
As we know all angles (interior) are equal in a polygon we can write:
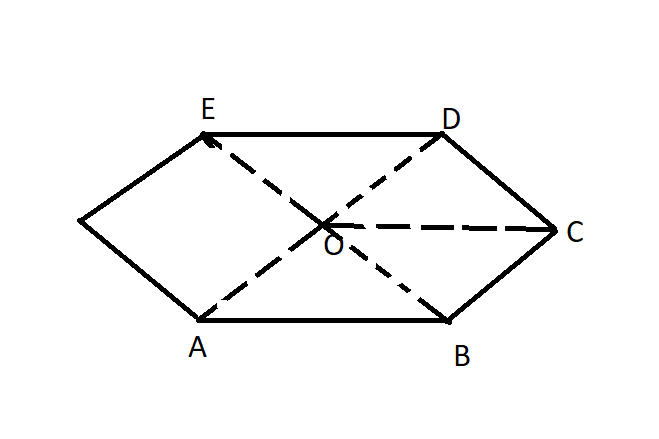
Let us take a n-sided polygon ABCDE. Take any point O inside and join OA, OB, OC,… For n-sided polygons, we get n triangles. We know sum of angles of triangles is
Sum of all interior angles + Sum of angles of angles at O
By diagram, we can say: Sum of angles at O
By substituting this and subtracting 360 on both sides, we get:
Sum of all interior angles
By substituting this in equation (1), we get:
Interior angle of polygon
We know the relation between exterior angle, interior angle.

They form a straight line. So, we say:
Exterior angle
By substituting equation (2), we can write the equation:
Exterior angle
By subtracting term on both sides, we can write it as:
Exterior angle
By simplifying the above equation, we can write it as:
Exterior angle
By multiplying with 8 on both sides, we get it as:
Given that each interior angle is 8 times the exterior.
By using elimination method, we can equate both equations (2), (3), we get it as:
By writing left hand side as 2 separate fractions we get it as:
By multiplying n on both sides, we get equation in form of:
By multiplying n inside the bracket, we get it in form:
By simplifying the above equation, we can write it as:
By adding 360 on both sides, we get it as:
By taking 360 as common, we get it in the form:
By simplifying the above equation, we can write it as:
By dividing with 180 on both sides, we get it as:
By simplifying above equation, we can write it as:
So, there are 18 sides for such a polygon given in question.
Therefore, option (c) is correct for the given question.
Note: We kept the term
Complete step-by-step answer:
As we know all angles (interior) are equal in a polygon we can write:

Let us take a n-sided polygon ABCDE. Take any point O inside and join OA, OB, OC,… For n-sided polygons, we get n triangles. We know sum of angles of triangles is
Sum of all interior angles + Sum of angles of angles at O
By diagram, we can say: Sum of angles at O
By substituting this and subtracting 360 on both sides, we get:
Sum of all interior angles
By substituting this in equation (1), we get:
Interior angle of polygon
We know the relation between exterior angle, interior angle.

They form a straight line. So, we say:
Exterior angle
By substituting equation (2), we can write the equation:
Exterior angle
By subtracting term on both sides, we can write it as:
Exterior angle
By simplifying the above equation, we can write it as:
Exterior angle
By multiplying with 8 on both sides, we get it as:
Given that each interior angle is 8 times the exterior.
By using elimination method, we can equate both equations (2), (3), we get it as:
By writing left hand side as 2 separate fractions we get it as:
By multiplying n on both sides, we get equation in form of:
By multiplying n inside the bracket, we get it in form:
By simplifying the above equation, we can write it as:
By adding 360 on both sides, we get it as:
By taking 360 as common, we get it in the form:
By simplifying the above equation, we can write it as:
By dividing with 180 on both sides, we get it as:
By simplifying above equation, we can write it as:
So, there are 18 sides for such a polygon given in question.
Therefore, option (c) is correct for the given question.
Note: We kept the term
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




