
Show that the function
Answer
538.8k+ views
Hint: To check the differentiability of a function, we will find the derivative of the function. To find the derivative of any function
Complete step-by-step answer:
In this question, we are given a function;
Since we have to check for the differentiability of this function, we have to first find its derivative. To find the derivative of the function
According to first principle, we can find derivative
Substituting
In trigonometry, we have a formula;
Substituting this formula i.e.
Since the limit can be distributed over subtraction of the two functions, we can write;
Since the limit is applied on
We have two formulas of limit,
Substituting
It will be easier to check the differentiability of
Plotting the graph of
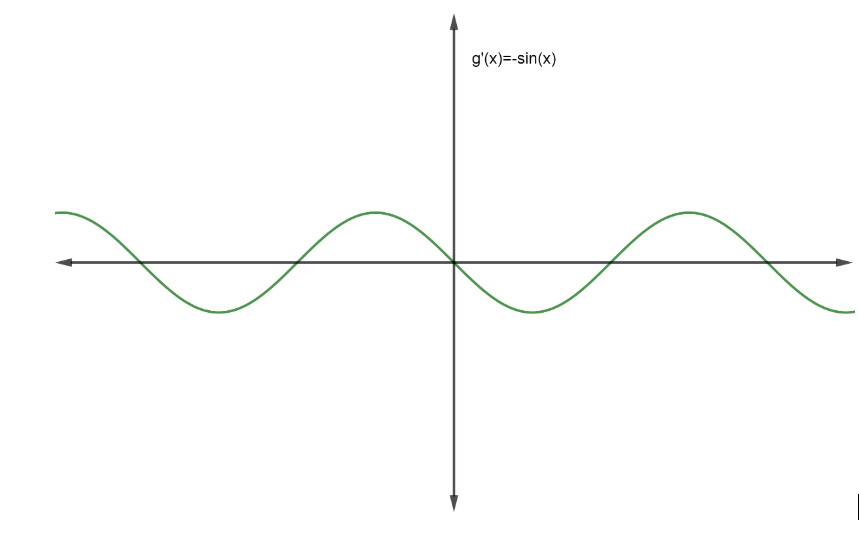
Since the graph of
Also,
Note: The question can be done directly if one has remembered that the derivatives of
Complete step-by-step answer:
In this question, we are given a function;
Since we have to check for the differentiability of this function, we have to first find its derivative. To find the derivative of the function
According to first principle, we can find derivative
Substituting
In trigonometry, we have a formula;
Substituting this formula i.e.
Since the limit can be distributed over subtraction of the two functions, we can write;
Since the limit is applied on
We have two formulas of limit,
Substituting
It will be easier to check the differentiability of
Plotting the graph of

Since the graph of
Also,
Note: The question can be done directly if one has remembered that the derivatives of
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Trending doubts
How many moles and how many grams of NaCl are present class 11 chemistry CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

Plants which grow in shade are called A Sciophytes class 11 biology CBSE

A renewable exhaustible natural resource is A Petroleum class 11 biology CBSE

In which of the following gametophytes is not independent class 11 biology CBSE

Find the molecular mass of Sulphuric Acid class 11 chemistry CBSE




