
Prove the following:
Answer
528k+ views
Hint: We will be using the concepts of trigonometric function to solve the problem. We will be using the trigonometric formulae like:
Complete step-by-step answer:
Now, we have to prove that,
Now, we know that for trigonometric ratios of supplementary angles are determined as,
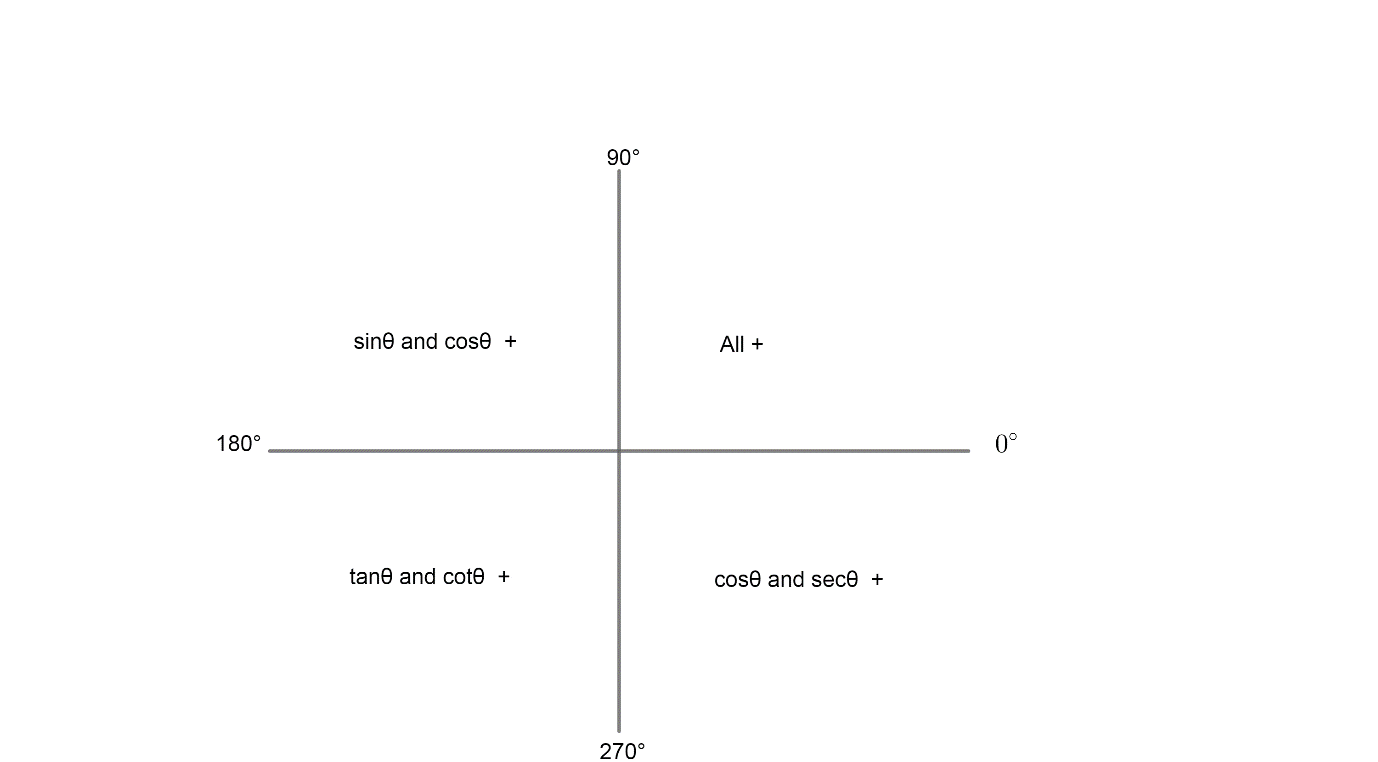
The figure shows the quadrant in which the trigonometric ratios are positive.
Now, it is important to note that the trigonometric ratio of an angle and its supplement is the same in magnitude but the sign depends on the quadrant in which it lies. For example:
Now, we take LHS,
Now, we have,
So, the LHS become,
Now, we know that
Therefore, we have,
Now, since LHS = RHS
Hence proved.
Note: To solve these type of questions it is important to note that we have used the ASTC quadrant system in which all the trigonometric ratios are positive in the first quadrant whereas only sine, cosec is positive in the second quadrant and tan, cot is positive in the third quadrant and cos, sec is positive in the fourth quadrant like
also it is important to remember the trigonometric formula like:
Hence, remembering these formulae will help to shorten the solution.
Complete step-by-step answer:
Now, we have to prove that,
Now, we know that for trigonometric ratios of supplementary angles are determined as,

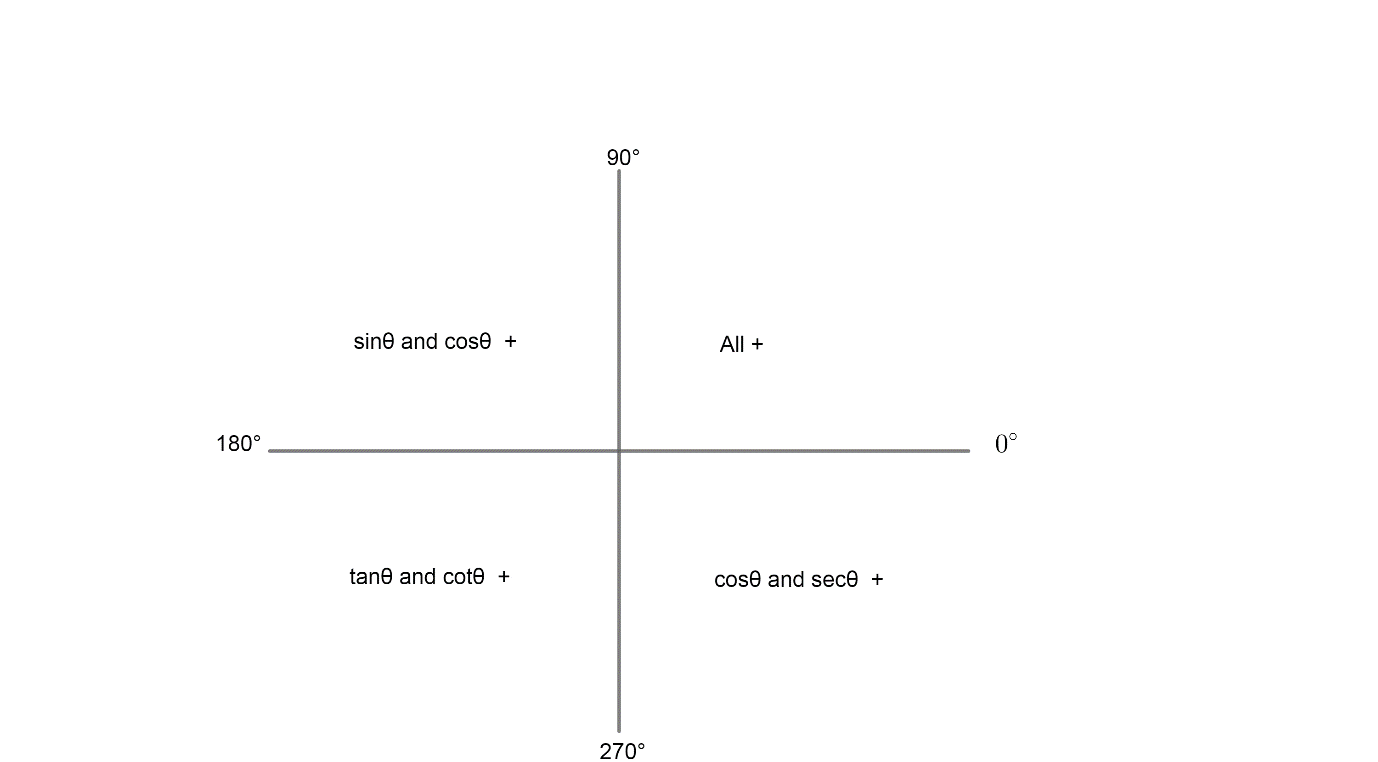
The figure shows the quadrant in which the trigonometric ratios are positive.
Now, it is important to note that the trigonometric ratio of an angle and its supplement is the same in magnitude but the sign depends on the quadrant in which it lies. For example:
Now, we take LHS,
Now, we have,
So, the LHS become,
Now, we know that
Therefore, we have,
Now, since LHS = RHS
Hence proved.
Note: To solve these type of questions it is important to note that we have used the ASTC quadrant system in which all the trigonometric ratios are positive in the first quadrant whereas only sine, cosec is positive in the second quadrant and tan, cot is positive in the third quadrant and cos, sec is positive in the fourth quadrant like

also it is important to remember the trigonometric formula like:
Hence, remembering these formulae will help to shorten the solution.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

The non protein part of an enzyme is a A Prosthetic class 11 biology CBSE

Which of the following blood vessels in the circulatory class 11 biology CBSE

What is a zygomorphic flower Give example class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

The deoxygenated blood from the hind limbs of the frog class 11 biology CBSE




