
Prove that the rhombus with equal diagonals is a square.
Answer
538.2k+ views
Hint:Draw a figure of rhombus when diagonals are equal. In rhombus, the diagonals bisect each other at right angles. Prove that the vertices are also right angles.
Complete step by step answer:
From the figure, let us say that ABCD is a rhombus.
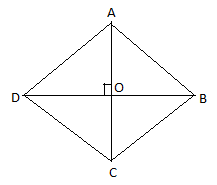
Let AC and BD be the diagonals of the rhombus. It is said that the rhombus has equal diagonals, i.e. AC = BD.
We know that diagonals of rhombus bisect each other. Thus we can say that AC = BD.
If AC = BD, then
AO = BO = CO = DO…..(1)
Consider the point where the diagonals intersect at O.
Let us consider the
From equation (1) we can say that AO = BO and
In rhombus, the diagonals bisect each other at right angles. Each diagonal cuts the other into two equal parts and the angle where they cross is always a right angle.
Thus we can say that
Similarly in
Thus from the figure,
Similarly, from the figure,
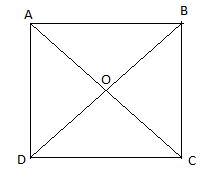
All the sides are also equal, i.e. AB = BC = CD = DA.
Therefore, we proved that a rhombus with equal diagonals is a square.
Note:In case of a square, we know the general properties, that all sides of a square are equal in length. Thus the diagonals of a square are equal in length and diagonals bisect each other. The angles are
Complete step by step answer:
From the figure, let us say that ABCD is a rhombus.


Let AC and BD be the diagonals of the rhombus. It is said that the rhombus has equal diagonals, i.e. AC = BD.
We know that diagonals of rhombus bisect each other. Thus we can say that AC = BD.
If AC = BD, then
AO = BO = CO = DO…..(1)
Consider the point where the diagonals intersect at O.
Let us consider the
From equation (1) we can say that AO = BO and
In rhombus, the diagonals bisect each other at right angles. Each diagonal cuts the other into two equal parts and the angle where they cross is always a right angle.
Thus we can say that
Similarly in
Thus from the figure,
Similarly, from the figure,
All the sides are also equal, i.e. AB = BC = CD = DA.
Therefore, we proved that a rhombus with equal diagonals is a square.
Note:In case of a square, we know the general properties, that all sides of a square are equal in length. Thus the diagonals of a square are equal in length and diagonals bisect each other. The angles are
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which of the following districts of Rajasthan borders class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

What is the full form of pH?

Define human made resources

India has states and Union Territories A 29 7 B 28 class 9 social science CBSE

What is the importance of natural resources? Why is it necessary to conserve them?




