
Prove that the diagonals of a parallelogram bisect each other.
Answer
499.5k+ views
Hint: We here have to prove that the diagonals of a parallelogram bisect each other. For this, we will first assume there to be a parallelogram ABCD whose diagonals will intersect each other at O. Then, we will see that as a result, 4 small triangles will be formed. Then we will consider any two opposite pairs of triangles and try to prove that they are congruent. Once they're proved to be congruent, the lines AO and OC will come out as equal and so will BO and OD as a result of CPCT rule. Hence, the diagonals will bisect each other.
Complete step by step answer:
We have to prove that the diagonals of a parallelogram bisect each other. For this, let us first assume there to be a ||gm ABCD whose diagonals AC and BD intersect each other at O.
This figure is given as follows:
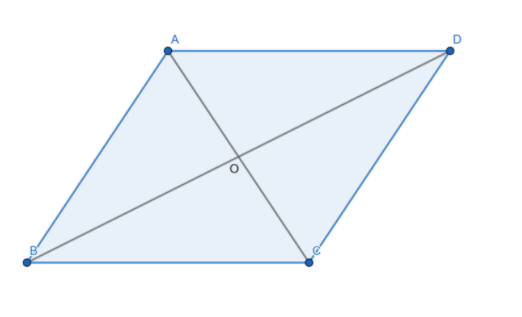
Now, if we consider
Side AB=side CD as they both are opposite sides of the parallelogram and we know that opposite sides of parallelogram are equal.
We will also see that
Thus, we can say that the triangles
Hence,
Now, if we apply CPCT (Corresponding Parts of Congruent Triangles), we will be able to see that:
AO=OC
BO=OD
Now, we can see from the figure that:
AO+OC=AC
BO+OD=BD
Thus, we can say that O is the midpoint of lines AC and BD.
Hence, the diagonals bisect each other at O.
Note: We here could have taken any two opposite pair of triangles and also could have used any congruence criteria to prove them congruent. This is shown as follows:
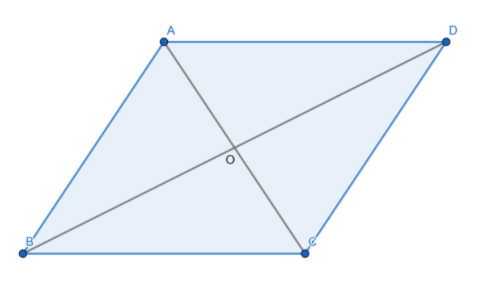
Let us consider
AD=BC as they are opposite sides of a parallelogram
Hence,
Complete step by step answer:
We have to prove that the diagonals of a parallelogram bisect each other. For this, let us first assume there to be a ||gm ABCD whose diagonals AC and BD intersect each other at O.
This figure is given as follows:

Now, if we consider
Side AB=side CD as they both are opposite sides of the parallelogram and we know that opposite sides of parallelogram are equal.
We will also see that
Thus, we can say that the triangles
Hence,
Now, if we apply CPCT (Corresponding Parts of Congruent Triangles), we will be able to see that:
AO=OC
BO=OD
Now, we can see from the figure that:
AO+OC=AC
BO+OD=BD
Thus, we can say that O is the midpoint of lines AC and BD.
Hence, the diagonals bisect each other at O.
Note: We here could have taken any two opposite pair of triangles and also could have used any congruence criteria to prove them congruent. This is shown as follows:

Let us consider
AD=BC as they are opposite sides of a parallelogram
Hence,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE




