
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Answer
442.2k+ views
1 likes
Hint: Draw a rough diagram of a quadrilateral circumscribing a circle. Join the centre of the circle will all the vertices of the quadrilateral. Consider any one vertex and using S – A – S (side – angle - side) congruence criteria prove that the line joining the vertex with the centre bisects that angle. Finally, use the property of the triangle that, “the sum of all interior angles of a triangle is
Complete step-by-step answer:
Let us draw a rough diagram of a quadrilateral circumscribing a circle.

In the above figure we have assumed a quadrilateral ABCD circumscribing a circle with centre O. the circle touches the sides AB, BC, CD and DA of the quadrilateral at P, Q, R and S respectively. So, these sides are behaving like tangents to the circle.
Here, we have joined OP and OS. We know that radius is perpendicular to the tangent at the point of contact. Therefore, OP and OS is perpendicular to AB and DA respectively.
Now, in right angle triangle OPA and OSA, we have,
OP = OS = radius of the circle
OA = OA = common sides
Therefore,
Similarly, we can prove the following three results: -
Let us draw the diagram with the following modifications in the assumptions.
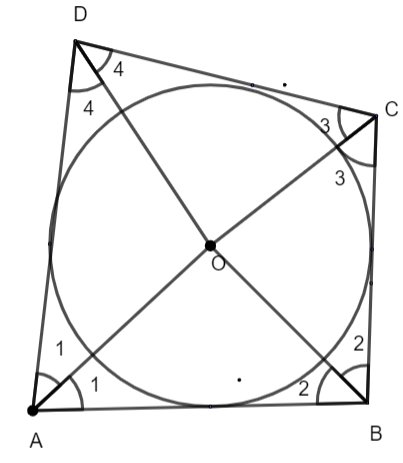
We know that the sum of interior angles of the quadrilateral is
Assume the above expression as equation (5).
Now, let us consider triangles AOB and COD. We have to prove that
And,
Adding equations (6) and (7), we get,
Using equation (5), we get,
Hence, we can say that the opposite sides of a quadrilateral circumscribing a circle subtend supplementary angle, i.e.
Similarly, we can prove the result for
Note: One may note that supplementary angles means the sum of angles must be
Complete step-by-step answer:
Let us draw a rough diagram of a quadrilateral circumscribing a circle.

In the above figure we have assumed a quadrilateral ABCD circumscribing a circle with centre O. the circle touches the sides AB, BC, CD and DA of the quadrilateral at P, Q, R and S respectively. So, these sides are behaving like tangents to the circle.
Here, we have joined OP and OS. We know that radius is perpendicular to the tangent at the point of contact. Therefore, OP and OS is perpendicular to AB and DA respectively.
Now, in right angle triangle OPA and OSA, we have,
OP = OS = radius of the circle
OA = OA = common sides
Therefore,
Similarly, we can prove the following three results: -
Let us draw the diagram with the following modifications in the assumptions.

We know that the sum of interior angles of the quadrilateral is
Assume the above expression as equation (5).
Now, let us consider triangles AOB and COD. We have to prove that
And,
Adding equations (6) and (7), we get,
Using equation (5), we get,
Hence, we can say that the opposite sides of a quadrilateral circumscribing a circle subtend supplementary angle, i.e.
Similarly, we can prove the result for
Note: One may note that supplementary angles means the sum of angles must be
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




