
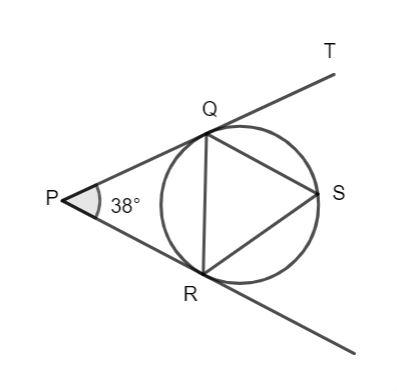
PQT and PR are tangents to the circle. If

Answer
537.9k+ views
1 likes
Hint: Find the angles PRQ and PQR using the properties of a tangent to the circle. Then find the angle RSQ, using the properties of the angle subtended by the chord. Then find angle RQS using properties of the triangle and finally find the angle TQS.
Complete Step-by-Step solution:
The tangent to the circle is the line that touches the circle only at one point.
The length of the tangents from the external point to the circle are equal.
Hence, the lengths of PQ and QR are equal to each other.
Hence, the triangle PQR is an isosceles triangle with side PQ equal to side PR, hence, the angle PQR and the angle PRQ are equal.
The value of angle QPR is given to be 38°.
The sum of angles of the triangle PQR is 180°. Then, we have:
Using equation (1), we have:
From equation (1), we have:
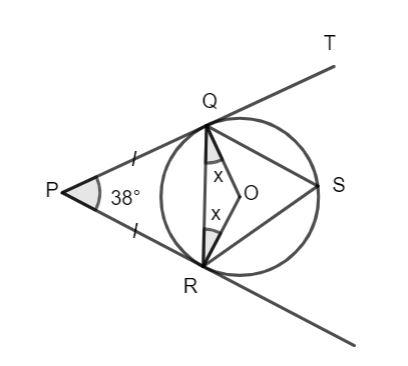
Let O be the center of the circle. The radius of the circle is always perpendicular to the tangent. Hence, OR is perpendicular to PR and OQ is perpendicular to PQ. Then, we have:
Using equation (3), we have:
The value of angle QOR in triangle ORQ is given as follows:
The angle inscribed by a chord on the circle is half of the angle subtended at the center. Hence, the value of angle QSR is half of the angle QOR.
It is given that angle PRS is equal to 111°.
Let's find angle SRQ.
Using equation (3), we have:
In triangle QRS, we have the sum of angles at 180°.
Using equations (4) and (5), we have:
PQT is a straight line, hence, the sum of the angles PQR, SQR, and TQS is 180°.
From equations (2) and (6), we have:
Hence, the value of angle TQS is 40°.
Note: Note that, in the figure, the angle QRS looks like it is equal to RQS but it is not. You need to find them individually using the sum of angles of the triangles.
Complete Step-by-Step solution:

The tangent to the circle is the line that touches the circle only at one point.
The length of the tangents from the external point to the circle are equal.
Hence, the lengths of PQ and QR are equal to each other.
Hence, the triangle PQR is an isosceles triangle with side PQ equal to side PR, hence, the angle PQR and the angle PRQ are equal.
The value of angle QPR is given to be 38°.
The sum of angles of the triangle PQR is 180°. Then, we have:
Using equation (1), we have:
From equation (1), we have:
Let O be the center of the circle. The radius of the circle is always perpendicular to the tangent. Hence, OR is perpendicular to PR and OQ is perpendicular to PQ. Then, we have:
Using equation (3), we have:
The value of angle QOR in triangle ORQ is given as follows:
The angle inscribed by a chord on the circle is half of the angle subtended at the center. Hence, the value of angle QSR is half of the angle QOR.
It is given that angle PRS is equal to 111°.
Let's find angle SRQ.
Using equation (3), we have:
In triangle QRS, we have the sum of angles at 180°.
Using equations (4) and (5), we have:
PQT is a straight line, hence, the sum of the angles PQR, SQR, and TQS is 180°.
From equations (2) and (6), we have:
Hence, the value of angle TQS is 40°.
Note: Note that, in the figure, the angle QRS looks like it is equal to RQS but it is not. You need to find them individually using the sum of angles of the triangles.
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




