
What is the number of atoms (z) in a simple cubic unit cell?
Answer
445.5k+ views
Hint :In a simple cubic unit cell, atoms are present only at the corners of the cube. Using this information, and the contribution of each atom to that particular unit cell, we can calculate the number of atoms in a simple cubic unit cell. A primitive or simple unit cell has motifs at the corners of the unit cell, except for in the case of a trigonal unit cell. Each crystal system has a primitive unit cell. Thus, there are a total of
Complete Step By Step Answer:
The three Bravais lattices belonging to the cubic system are simple (P), body-centred (I) and face-centred (F).
The simple cubic unit cell can be depicted as follows:
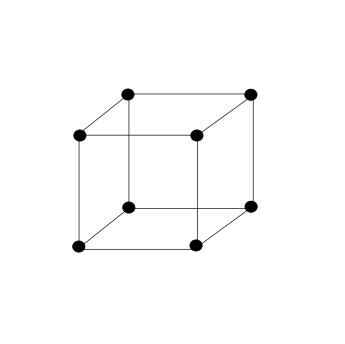
As shown in the picture, there are atoms only at the corners of the unit cell. A cube has
Hence, the number of atoms (z) present in a simple cubic unit cell is
Note :
In a body-centred cubic unit cell, there atoms at the
Complete Step By Step Answer:
The three Bravais lattices belonging to the cubic system are simple (P), body-centred (I) and face-centred (F).
The simple cubic unit cell can be depicted as follows:

As shown in the picture, there are atoms only at the corners of the unit cell. A cube has
Hence, the number of atoms (z) present in a simple cubic unit cell is
Note :
In a body-centred cubic unit cell, there atoms at the
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why should a magnesium ribbon be cleaned before burning class 12 chemistry CBSE

Mirage is a phenomena due to A Refraction of light class 12 physics CBSE

Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

How is democracy better than other forms of government class 12 social science CBSE

What is Nutrition Explain Diff Type of Nutrition ?




