
Multiply
Answer
508.2k+ views
Hint: In this question we have to multiply the given terms by using the lattice method. For this first we have to know what the lattice method is.
Lattice Method:
We first calculate a product for each cell by multiplying the digit at the top of the column and the digit at the right of the row. The tens digit of the product is placed above the diagonal that passes through the cell, and the units digit is put below that diagonal.
Multiplication by lattice method:
Write the numbers to be multiplied on the top and on the right.
Multiply the number on the top of the box with the number to the right of the box and write the answer in the box.
Add the numbers in the box diagonally and carry to the next column when necessary.
After the grid is completed, we will get the multiplication.
Complete step-by-step answer:
We need to multiply
Arrange
Multiply
Notice that
By the same token, multiply
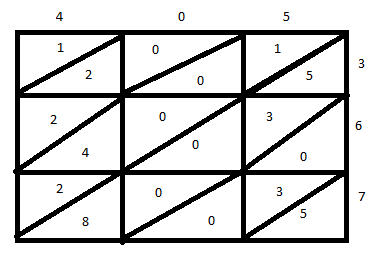
Then, going from right to left, add the numbers down the diagonals.
The first diagonal has only
The second diagonal has
The third diagonal has
The fourth diagonal has
and so forth...
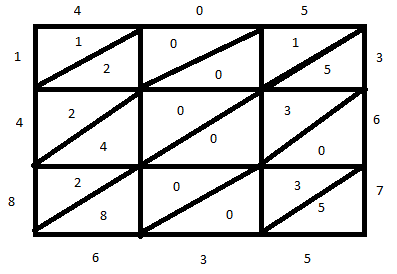
After the grid is completed, what we get is the answer to the multiplication that is
Note: Grids should be made properly. Take care while joining diagonals of squares. Or else our answer may go wrong. This is like our traditional method only. But in a much easier way. As in this method, the steps are broken & carried forward digits are written within the grids. We can be friendly with this method by more & more practice.
Lattice Method:
We first calculate a product for each cell by multiplying the digit at the top of the column and the digit at the right of the row. The tens digit of the product is placed above the diagonal that passes through the cell, and the units digit is put below that diagonal.
Multiplication by lattice method:
Write the numbers to be multiplied on the top and on the right.
Multiply the number on the top of the box with the number to the right of the box and write the answer in the box.
Add the numbers in the box diagonally and carry to the next column when necessary.
After the grid is completed, we will get the multiplication.
Complete step-by-step answer:
We need to multiply
Arrange

Multiply
Notice that
By the same token, multiply

Then, going from right to left, add the numbers down the diagonals.
The first diagonal has only
The second diagonal has
The third diagonal has
The fourth diagonal has
and so forth...

After the grid is completed, what we get is the answer to the multiplication that is
Note: Grids should be made properly. Take care while joining diagonals of squares. Or else our answer may go wrong. This is like our traditional method only. But in a much easier way. As in this method, the steps are broken & carried forward digits are written within the grids. We can be friendly with this method by more & more practice.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




