Question

Moment of inertia does not depend upon
A. Angular velocity of the body
B. Shape and size
C. Mass
D. Position of the axis of rotation

Answer 1

Hint: Moment of inertia of a body is defined as the quantity which is expressed by the body resisting the angular acceleration which is given as the product of the mass of every particle with its square of the distance from the axis of the rotation.

Complete step-by-step solution
The moment of inertia is defined as the quantity which decides the amount of torque needed for a specific angular acceleration in a rotational axis. It is also known as the angular mass or rotational inertia.
The moment of inertia of a body depends upon the following factors:
1) Density of the material
2) Shape and size of the body
3) Axis of the rotation
For the same mass and size of the solid body, it depends on how the mass is distributed relative to the axis (like the moment of inertia is always relative to a given axis). The farther the mass or a body is located from the axis, the bigger is the coefficient (so the larger moment of inertia for given mass and size of the body).

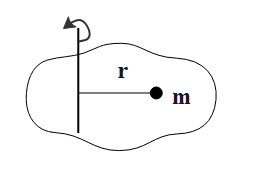
Here, let us say that the above body is a collection of small point masses. Let us suppose that the mass of the point mass is $m$and its distance from the axis is ‘r’. So, the moment of inertia of the mass $m$ is given as,
$I=m{r}^2$
From the given options we can say that the moment of inertia of a body is independent of the angular velocity of the body. Because we know that the moment of inertia of a body is the product of the mass of the body and the square of the distance of the body from the axis. So, it depends only upon the mass and distance of the mass from the axis.
Hence, option (A) is the correct answer.

Note: If we are considering a non-rotating body then, Inertia is the resistance to change in the state of motion. The more is mass the more it will resist change in its motion. Thus, we can say that it depends on the mass of the body.