
Let
A.
B.
C.
D.
Answer
512.4k+ views
Hint: Here we use the concept of odd functions which is
Complete step-by-step answer:
We have
Also, we know any function is an odd function if it satisfies
Now we have to find the value of the function at point
We have to find the value of
Since, f is an odd function, therefore, we can use the concept
Now we can break the angle inside the function as
Separating the fraction into two parts
Cancel out common factors from numerator and denominator
Therefore, we can write
Now we know
Put
Now we will use the quadrant graph to convert the angles.
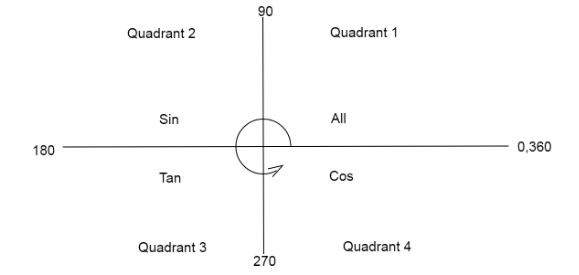
Here we denote
Now we calculate the values of both the functions on RHS of the equation using the quadrant diagram.
For
So, the value of
For
So, the value of
Substitute the values from equation (2) and equation (3) in equation (1)
Multiply the terms in the bracket.
So now
Therefore,
So, option B is correct.
Note: Students are likely to make mistakes while calculating the values from the quadrant diagram, keep in mind that we always move anti-clockwise as we add the angles, so when we subtract the angle we move backwards or clockwise to see which quadrant our function lies in.
Complete step-by-step answer:
We have
Also, we know any function is an odd function if it satisfies
Now we have to find the value of the function at point
We have to find the value of
Since, f is an odd function, therefore, we can use the concept
Now we can break the angle inside the function as
Separating the fraction into two parts
Cancel out common factors from numerator and denominator
Therefore, we can write
Now we know
Put
Now we will use the quadrant graph to convert the angles.

Here we denote
Now we calculate the values of both the functions on RHS of the equation using the quadrant diagram.
For
So, the value of
For
So, the value of
Substitute the values from equation (2) and equation (3) in equation (1)
Multiply the terms in the bracket.
So now
Therefore,
So, option B is correct.
Note: Students are likely to make mistakes while calculating the values from the quadrant diagram, keep in mind that we always move anti-clockwise as we add the angles, so when we subtract the angle we move backwards or clockwise to see which quadrant our function lies in.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




