
How do inflection points differ from critical points?
Answer
478.8k+ views
Hint: These types of theoretical questions are very easy to solve. We just need to understand the main underlying concept. In this question we first need to understand what inflection points and critical points are. Inflection points are those which determine the nature of the curve. Critical points are those which are generally used to determine the points of maxima or minima of the graph which will be given.
Complete step by step answer:
Now we start off the solution by saying that,
Let us assume that
Suppose we don’t find any critical points, then we have to find the second derivative and equating it to zero,
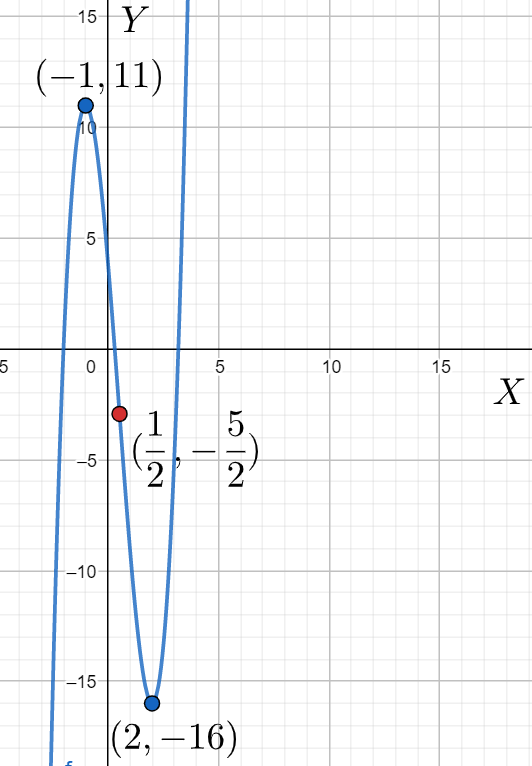
Suppose we consider an equation,
Note:For any given problem, we first have to read the problem very carefully to find what has been asked, to find the critical points of the inflection point. Whatever asked to find, we must follow the above steps serially to get the correct answer. We should also remember that the odd derivatives of a function always give us the critical points and the even derivatives give the inflection points.
Complete step by step answer:
Now we start off the solution by saying that,
Let us assume that
Suppose we don’t find any critical points, then we have to find the second derivative and equating it to zero,

Suppose we consider an equation,
Note:For any given problem, we first have to read the problem very carefully to find what has been asked, to find the critical points of the inflection point. Whatever asked to find, we must follow the above steps serially to get the correct answer. We should also remember that the odd derivatives of a function always give us the critical points and the even derivatives give the inflection points.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




