
In the given network of capacitors, the equivalent capacitance between point
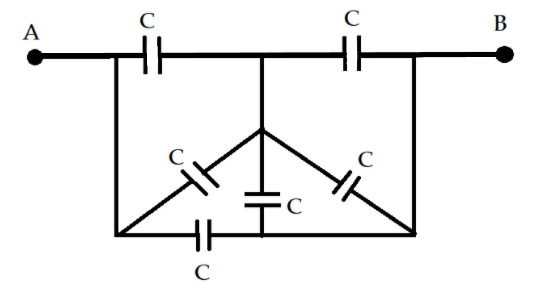
a.
b.
c.
d.

Answer
486.9k+ views
Hint: Here in this question capacitors are connected in different arrangements in a circuit between two given points. A capacitor is generally an electronic device that stores electrical energy in the electric field and it is a passive electronic component as it receives energy.
Formula used:
Parallel connection of the capacitors
Series connection of the capacitors
Complete step by step answer:
We will start with the different arrangements of the components and analyze whether which are connected in series connection or parallel connection and then they are calculated separately and then the equivalence capacitor is then evaluated.
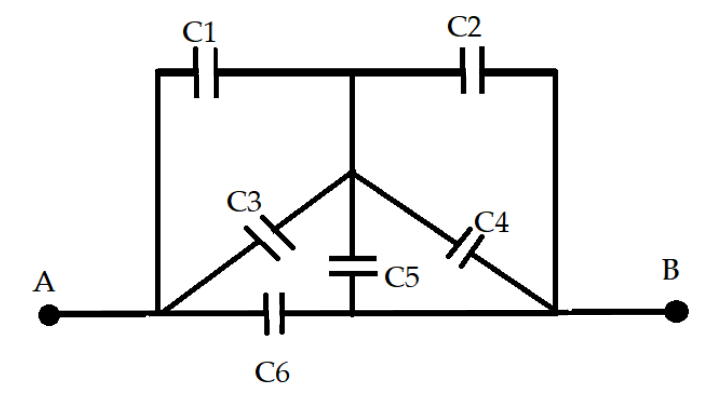
Staring with the rearrangements of the points
From the figure, it can be concluded that the capacitors
Substitute the values of
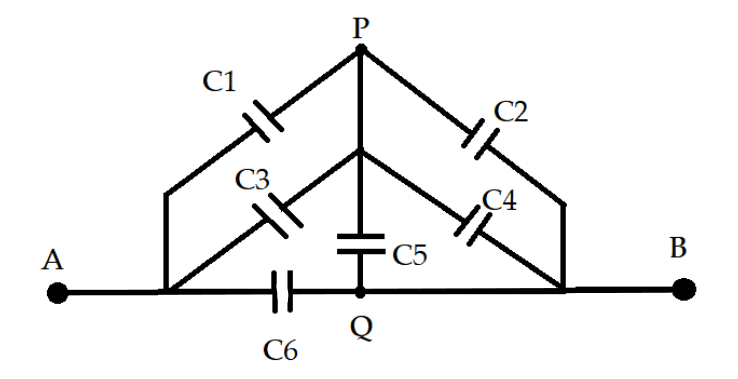
Similarly, the between points
Now on analyzing, it is found that the capacitor with
Hence the final equivalent capacitance between the points
Hence the final equivalent capacitance between the points
Hence, the correct answer is option (D).
Note: Here it is noted that the equivalence formula of capacitors is different from the other electronic components like resistance. Hence one should not get confused between the equivalence formula between resistance and capacitors. For resistance connected in series, the equivalence formula is given by
Formula used:
Parallel connection of the capacitors
Series connection of the capacitors
Complete step by step answer:
We will start with the different arrangements of the components and analyze whether which are connected in series connection or parallel connection and then they are calculated separately and then the equivalence capacitor is then evaluated.

Staring with the rearrangements of the points

From the figure, it can be concluded that the capacitors
Substitute the values of
Similarly, the between points
Now on analyzing, it is found that the capacitor with
Hence the final equivalent capacitance between the points
Hence the final equivalent capacitance between the points
Hence, the correct answer is option (D).
Note: Here it is noted that the equivalence formula of capacitors is different from the other electronic components like resistance. Hence one should not get confused between the equivalence formula between resistance and capacitors. For resistance connected in series, the equivalence formula is given by
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




