
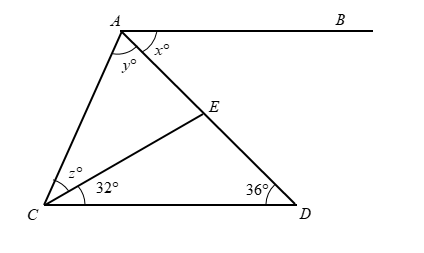
In the given figure,

Answer
490.8k+ views
1 likes
Hint: Here, we need to find the values of
Complete step-by-step answer:
We can observe that
Therefore, we get the alternate interior angles
Substituting
Thus, we get the value of
Next, it is given that
Therefore,
We know that in an isosceles triangle, the angles that are opposite to equal sides are equal.
Therefore, since
Substituting
Now, we will use the exterior angle property of a triangle.
In triangle
Substituting
Adding the terms in the expression, we get
Thus, we get the value of
Therefore, we get
Finally, we will use the angle sum property of a triangle to get the value of
Using the angle sum property in triangle
Substituting
Adding the terms of the expression, we get
Subtracting
Thus, we get the value of
Therefore, the values of
Note: We can also calculate the value of
The co-interior angles on the same side of a transversal, intersecting two parallel lines, are supplementary.
We can observe that
Therefore, we get
Rewriting
Substituting
Adding the terms of the expression, we get
Subtracting
Thus, we get the value of
Complete step-by-step answer:
We can observe that
Therefore, we get the alternate interior angles
Substituting
Thus, we get the value of
Next, it is given that
Therefore,
We know that in an isosceles triangle, the angles that are opposite to equal sides are equal.
Therefore, since
Substituting
Now, we will use the exterior angle property of a triangle.
In triangle
Substituting
Adding the terms in the expression, we get
Thus, we get the value of
Therefore, we get
Finally, we will use the angle sum property of a triangle to get the value of
Using the angle sum property in triangle
Substituting
Adding the terms of the expression, we get
Subtracting
Thus, we get the value of
Therefore, the values of
Note: We can also calculate the value of
The co-interior angles on the same side of a transversal, intersecting two parallel lines, are supplementary.
We can observe that
Therefore, we get
Rewriting
Substituting
Adding the terms of the expression, we get
Subtracting
Thus, we get the value of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE




