Answer
393.9k+ views
Hint: We will first join A and B, then prove the triangles ODA and OCD to be congruent and triangles OCE and OBE to be congruent and then consider the angles associated with line AB to get the required result.
Complete step-by-step answer:
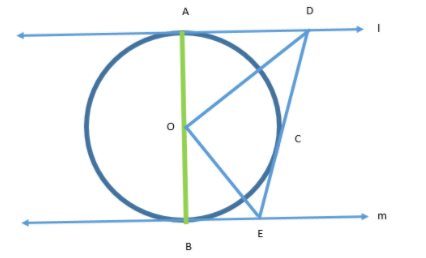
Let us first join A and B as follows:
Now, let us consider $\vartriangle OAD$ and $\vartriangle OCD$:
OA = OC (Radii of the same circle)
OD = OD (Common)
AD = CD (Tangents from same external point are equal to each other)
(A line which touches a circle or ellipse at just one point is known as a tangent)
Hence, $\vartriangle OAD \cong \vartriangle OCD$ (By SSS property)
(The SSS rule states that: If three sides of one triangle are equal to three sides of another triangle, then the triangles are congruent.)
Therefore, $\angle AOD = \angle COD$ (BY CPCT) ………….(1)
(CPCT stands for Corresponding parts of congruent triangles. CPCT theorem states that if two or more triangles which are congruent to each other are taken then the corresponding angles and the sides of the triangles are also congruent to each other.)
Now, if we do the same process in $\vartriangle OBE$ and $\vartriangle OCE$, we will get:
$\vartriangle OBE \cong \vartriangle OCE$(By SSS property)
Now, using CPCT, we will get:- $\angle BOE = \angle COE$ ………….(2)
We are already given that O is the center and since AB passes through O and touches the boundary of the circle. Hence, AB is a diameter.
Hence, it is a straight line.
Therefore, \[\angle AOB = {180^ \circ }\]
We can rewrite it as:-
$\angle AOD + \angle DOC + \angle COE + \angle EOB = {180^ \circ }$
Now using (1) and (2), we can rewrite it as:-
$\angle COD + \angle DOC + \angle COE + \angle COE = {180^ \circ }$
$ \Rightarrow 2\angle COD + 2\angle COE = {180^ \circ }$
$ \Rightarrow 2(\angle COD + \angle COE) = {180^ \circ }$
$ \Rightarrow 2(\angle DOE) = {180^ \circ }$
$ \Rightarrow \angle DOE = {90^ \circ }$.
Hence, proved.
Note: The students must remember that they need to write the reasons for the equations they are using to prove some triangles congruent. Otherwise, the answer could be considered vague. Students must note that they need to prove that we have a straight line to use the sum of angles to be linear.
Complete step-by-step answer:
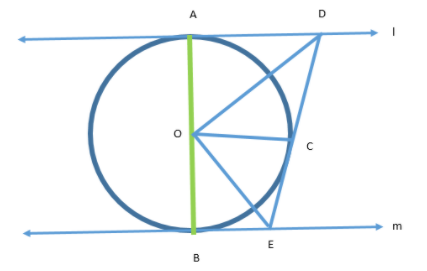
Let us first join A and B as follows:

Now, let us consider $\vartriangle OAD$ and $\vartriangle OCD$:
OA = OC (Radii of the same circle)
OD = OD (Common)
AD = CD (Tangents from same external point are equal to each other)
(A line which touches a circle or ellipse at just one point is known as a tangent)
Hence, $\vartriangle OAD \cong \vartriangle OCD$ (By SSS property)
(The SSS rule states that: If three sides of one triangle are equal to three sides of another triangle, then the triangles are congruent.)
Therefore, $\angle AOD = \angle COD$ (BY CPCT) ………….(1)
(CPCT stands for Corresponding parts of congruent triangles. CPCT theorem states that if two or more triangles which are congruent to each other are taken then the corresponding angles and the sides of the triangles are also congruent to each other.)
Now, if we do the same process in $\vartriangle OBE$ and $\vartriangle OCE$, we will get:
$\vartriangle OBE \cong \vartriangle OCE$(By SSS property)
Now, using CPCT, we will get:- $\angle BOE = \angle COE$ ………….(2)
We are already given that O is the center and since AB passes through O and touches the boundary of the circle. Hence, AB is a diameter.
Hence, it is a straight line.
Therefore, \[\angle AOB = {180^ \circ }\]
We can rewrite it as:-
$\angle AOD + \angle DOC + \angle COE + \angle EOB = {180^ \circ }$
Now using (1) and (2), we can rewrite it as:-
$\angle COD + \angle DOC + \angle COE + \angle COE = {180^ \circ }$
$ \Rightarrow 2\angle COD + 2\angle COE = {180^ \circ }$
$ \Rightarrow 2(\angle COD + \angle COE) = {180^ \circ }$
$ \Rightarrow 2(\angle DOE) = {180^ \circ }$
$ \Rightarrow \angle DOE = {90^ \circ }$.
Hence, proved.
Note: The students must remember that they need to write the reasons for the equations they are using to prove some triangles congruent. Otherwise, the answer could be considered vague. Students must note that they need to prove that we have a straight line to use the sum of angles to be linear.
Recently Updated Pages
Three beakers labelled as A B and C each containing 25 mL of water were taken A small amount of NaOH anhydrous CuSO4 and NaCl were added to the beakers A B and C respectively It was observed that there was an increase in the temperature of the solutions contained in beakers A and B whereas in case of beaker C the temperature of the solution falls Which one of the following statements isarecorrect i In beakers A and B exothermic process has occurred ii In beakers A and B endothermic process has occurred iii In beaker C exothermic process has occurred iv In beaker C endothermic process has occurred

The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Trending doubts
Assertion CNG is a better fuel than petrol Reason It class 11 chemistry CBSE

How does pressure exerted by solid and a fluid differ class 8 physics CBSE

Number of valence electrons in Chlorine ion are a 16 class 11 chemistry CBSE

What are agricultural practices? Define

What does CNG stand for and why is it considered to class 10 chemistry CBSE

The rate of evaporation depends on a Surface area b class 9 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

State whether the following statement is true or false class 11 physics CBSE

A night bird owl can see very well in the night but class 12 physics CBSE