
In the direction of electric field, the electric potential:
A. Decreases.
B. Increases.
C. Remains unchanged.
D. Becomes zero.
Answer
534k+ views
Hint: We will use the idea of the electric field as a gradient of potential to answer this problem. We are going to solve this problem using the relationship
Complete step by step answer:
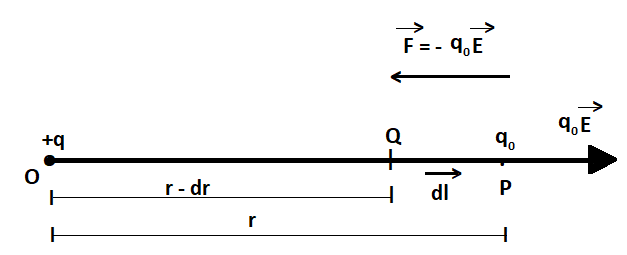
We know that in an electrical field the electrical potential between two points A and B is defined as the amount of work performed per unit of positive test charge in moving it against the electrostatic force due to the electrical field from point A to point B.
If
And, if
Therefore, work done to move the test charge through an infinitely small displacement
and we have
As the distance r decreases in the direction of
So now, form equation (ii), we get
From equation (i), we have
Therefore the electric field at a point is equal to the negative gradient of the electric potential at that point.
The negative sign indicates that the direction of E is always in the direction of decreased potential.
Hence, we can say that, In the direction of electric field, the electric potential decreases.
Therefore the correct answer is option (A).
Note: In this type of questions, first we have to identify what relationship of the given terms is required to solve the question. Then we will assume some conditions and prove the statement step by step. While solving the question, we have to remember the significance of the signs. After that we will get the required answer.
Complete step by step answer:

We know that in an electrical field the electrical potential between two points A and B is defined as the amount of work performed per unit of positive test charge in moving it against the electrostatic force due to the electrical field from point A to point B.
If
And, if
Therefore, work done to move the test charge through an infinitely small displacement
and we have
As the distance r decreases in the direction of
So now, form equation (ii), we get
From equation (i), we have
Therefore the electric field at a point is equal to the negative gradient of the electric potential at that point.
The negative sign indicates that the direction of E is always in the direction of decreased potential.
Hence, we can say that, In the direction of electric field, the electric potential decreases.
Therefore the correct answer is option (A).
Note: In this type of questions, first we have to identify what relationship of the given terms is required to solve the question. Then we will assume some conditions and prove the statement step by step. While solving the question, we have to remember the significance of the signs. After that we will get the required answer.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

When and how did Canada eventually gain its independence class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




