
In figure ABCDE, find


Answer
477.6k+ views
2 likes
Hint: The angles in the sum given in the above question are the interior angles of the pentagon. To find the sum, we need to use the property that the sum of all the exterior angles of a polygon is always equal to
Complete step-by-step solution:
Let us consider all of the exterior angles of the pentagon given in the above question in the below figure.
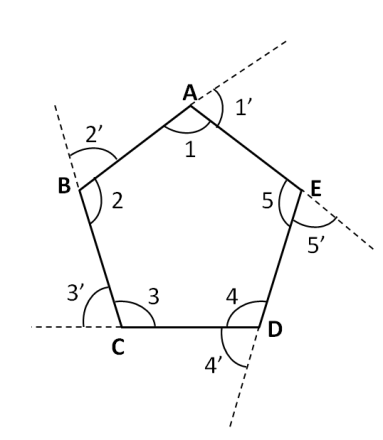
Now, we know that the sum of the exterior angles of any polygon is equal to
Now, as can be seen in the above figure, the angles
Similarly, we can have the following equations
Adding the equations (ii), (iii), (iv), (v), and (vi) we get
Substituting the equation (i) in the above equation, we get
Hence, the given sum
Note: We can also solve this question by using the formula for the sum of the interior angles of a polygon, which is given by
Complete step-by-step solution:
Let us consider all of the exterior angles of the pentagon given in the above question in the below figure.

Now, we know that the sum of the exterior angles of any polygon is equal to
Now, as can be seen in the above figure, the angles
Similarly, we can have the following equations
Adding the equations (ii), (iii), (iv), (v), and (vi) we get
Substituting the equation (i) in the above equation, we get
Hence, the given sum
Note: We can also solve this question by using the formula for the sum of the interior angles of a polygon, which is given by
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
The Chinese traveller FaHien visited India and left class 8 social science CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

What is 1 divided by 0 class 8 maths CBSE

How many ounces are in 500 mL class 8 maths CBSE

Name the states through which the Tropic of Cancer class 8 social science CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE




