
In a series RLC circuit that is operating above the resonant frequency, the current
A. lags the applied voltage
B. leads the applied voltage
C. is in phase with the applied voltage
D. is zero
Answer
490.8k+ views
1 likes
Hint: At resonance the capacitive reactance and inductive reactance is equal. At above the resonant frequency the inductive reactance will be greater than the capacitive reactance. So a RLC circuit operating above resonant frequency behaves as a purely inductive circuit. The phase difference between the current and voltage will be the same in this circuit as the inductive circuit.
Formulas used:
Complete answer:
For a circuit containing resistor, capacitor, and inductor
The inductive reactance is given by
Where
And the capacitive reactance is given by
Current flowing through LCR circuit is given by
Where Z is impedance. At resonance Z will be equal to R only.
We know that inductive circuit current lags voltage and in capacitive circuit current leads voltage.
If we draw plot of current passing through LCR circuit VS input frequency it will exactly look like the below diagram
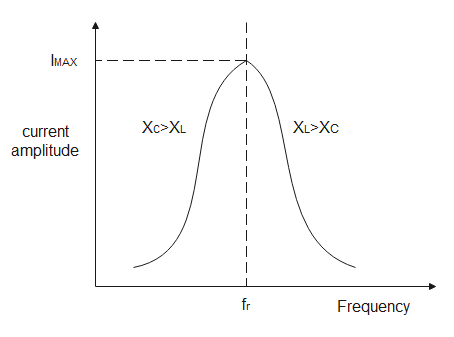
When capacitive reactance equals inductive reactance then that condition is called resonance.
At resonance
Where
In the region where operating frequency is greater than resonating frequency, as per the diagram
Hence option A is correct.
Note:
If we clearly observe the above diagram, at resonating frequency current flowing is maximum because impedance which is resultant of capacitive reactance and inductive reactance and resistance is minimum there. When impedance is minimum, the current flowing will be maximum. Voltage across resistors is always in phase with current. So we are never concerned about the phase difference between the resistor voltage and its current.
Formulas used:
Complete answer:
For a circuit containing resistor, capacitor, and inductor
The inductive reactance is given by
Where
And the capacitive reactance is given by
Current flowing through LCR circuit is given by
Where Z is impedance. At resonance Z will be equal to R only.
We know that inductive circuit current lags voltage and in capacitive circuit current leads voltage.
If we draw plot of current passing through LCR circuit VS input frequency it will exactly look like the below diagram

When capacitive reactance equals inductive reactance then that condition is called resonance.
At resonance
Where
In the region where operating frequency is greater than resonating frequency, as per the diagram
Hence option A is correct.
Note:
If we clearly observe the above diagram, at resonating frequency current flowing is maximum because impedance which is resultant of capacitive reactance and inductive reactance and resistance is minimum there. When impedance is minimum, the current flowing will be maximum. Voltage across resistors is always in phase with current. So we are never concerned about the phase difference between the resistor voltage and its current.
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




