Answer
356.3k+ views
Hint: Here, we need to find the measure of two acute angles. To solve the question, we will assume the two acute angles to be \[2x\] and \[3x\] respectively. We will apply the angle sum property to form an equation in terms of \[x\]. We will solve the equation to find the value of \[x\] and then using its value we will find the measure of the two acute angles.
Complete step-by-step answer:
We will use the angle sum property of a triangle to find the measure of the angles of the triangle.
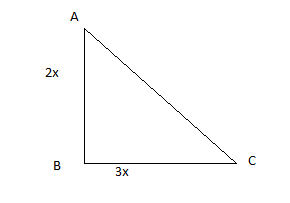
It is given that the two acute angles are in the ratio \[2:3\].
Let the two acute angles be \[2x\] and \[3x\] respectively.
As we know, the third angle of the triangle is a right angle.
Thus, the measure of the third angle is \[90^\circ \].
Now, the angle sum property of a triangle states that the sum of the measures of the three interior angles of a triangle is always \[180^\circ \].
Thus, the sum of the two acute angles and the right angle will be equal to \[180^\circ \].
Therefore, we get
\[2x + 3x + 90^\circ = 180^\circ \]
We will now solve the equation to find the value of \[x\].
Subtracting \[90^\circ \] from both sides of the equation, we get
\[\begin{array}{l} \Rightarrow 2x + 3x + 90^\circ - 90^\circ = 180^\circ - 90^\circ \\ \Rightarrow 2x + 3x = 90^\circ \end{array}\]
Adding the like terms in the equation, we get
\[ \Rightarrow 5x = 90^\circ \]
Dividing both sides by 5, we get
\[\begin{array}{l} \Rightarrow \dfrac{{5x}}{5} = \dfrac{{90^\circ }}{5}\\ \Rightarrow x = 18^\circ \end{array}\]
Therefore, we get the value of \[x\] as \[18^\circ \].
Finally, we will substitute the value of \[x\] to find the measures of the two acute angles.
Substituting \[x = 18^\circ \] in \[2x\], we get the first acute angle as
\[2x = 2 \times 18^\circ = 36^\circ \]
Substituting \[x = 18^\circ \] in \[3x\], we get the second acute angle as
\[3x = 3 \times 18^\circ = 54^\circ \]
\[\therefore\] The measure of the two acute angles of the right angled triangle are \[36^\circ \] and \[54^\circ \] respectively.
Note: It is given in the question that the triangle is right angled, which means one of the angles of the triangle is \[90^\circ \]. If it was given an equilateral triangle, it means that every angle of the triangle is \[60^\circ \]. We need to also keep in mind that the sum of interior angles of a triangle is \[180^\circ \] and not \[360^\circ \] which is the sum of interior angles of a quadrilateral.
Complete step-by-step answer:
We will use the angle sum property of a triangle to find the measure of the angles of the triangle.
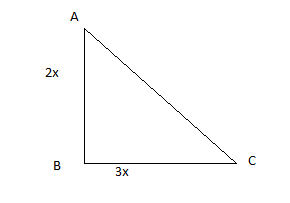
It is given that the two acute angles are in the ratio \[2:3\].
Let the two acute angles be \[2x\] and \[3x\] respectively.
As we know, the third angle of the triangle is a right angle.
Thus, the measure of the third angle is \[90^\circ \].
Now, the angle sum property of a triangle states that the sum of the measures of the three interior angles of a triangle is always \[180^\circ \].
Thus, the sum of the two acute angles and the right angle will be equal to \[180^\circ \].
Therefore, we get
\[2x + 3x + 90^\circ = 180^\circ \]
We will now solve the equation to find the value of \[x\].
Subtracting \[90^\circ \] from both sides of the equation, we get
\[\begin{array}{l} \Rightarrow 2x + 3x + 90^\circ - 90^\circ = 180^\circ - 90^\circ \\ \Rightarrow 2x + 3x = 90^\circ \end{array}\]
Adding the like terms in the equation, we get
\[ \Rightarrow 5x = 90^\circ \]
Dividing both sides by 5, we get
\[\begin{array}{l} \Rightarrow \dfrac{{5x}}{5} = \dfrac{{90^\circ }}{5}\\ \Rightarrow x = 18^\circ \end{array}\]
Therefore, we get the value of \[x\] as \[18^\circ \].
Finally, we will substitute the value of \[x\] to find the measures of the two acute angles.
Substituting \[x = 18^\circ \] in \[2x\], we get the first acute angle as
\[2x = 2 \times 18^\circ = 36^\circ \]
Substituting \[x = 18^\circ \] in \[3x\], we get the second acute angle as
\[3x = 3 \times 18^\circ = 54^\circ \]
\[\therefore\] The measure of the two acute angles of the right angled triangle are \[36^\circ \] and \[54^\circ \] respectively.
Note: It is given in the question that the triangle is right angled, which means one of the angles of the triangle is \[90^\circ \]. If it was given an equilateral triangle, it means that every angle of the triangle is \[60^\circ \]. We need to also keep in mind that the sum of interior angles of a triangle is \[180^\circ \] and not \[360^\circ \] which is the sum of interior angles of a quadrilateral.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Why Are Noble Gases NonReactive class 11 chemistry CBSE

Let X and Y be the sets of all positive divisors of class 11 maths CBSE

Let x and y be 2 real numbers which satisfy the equations class 11 maths CBSE

Let x 4log 2sqrt 9k 1 + 7 and y dfrac132log 2sqrt5 class 11 maths CBSE

Let x22ax+b20 and x22bx+a20 be two equations Then the class 11 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

At which age domestication of animals started A Neolithic class 11 social science CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Write a letter to the principal requesting him to grant class 10 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Fill in the blanks A 1 lakh ten thousand B 1 million class 9 maths CBSE



