Question

If the tangent at a point on the ellipse $\dfrac{{{x^2}}}{{27}} + \dfrac{{{y^2}}}{3} = 1$ meets the coordinate axes at A and B, and O is the origin, and then find the minimum area (in square units) of the triangle OAB.
(A) $\dfrac{9}{2}$
(B) $9$
(C) $3\sqrt 3 $
(D) $9\sqrt 3 $

Answer 1

Hint: Draw a rough diagram from the information given in the question. Now write the equation for the tangent of an ellipse in the parametric form at the point $\left( {a\cos \theta ,b\sin \theta } \right)$. Find the x and y-intercepts of this line. Form a triangle with the intercepts and origin and find out the minimum area of this triangle using trigonometric functions.

Complete step-by-step answer:

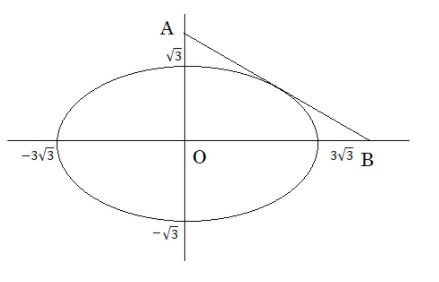
Here in the problem, we are given with an ellipse with an equation as $\dfrac{{{x^2}}}{{27}} + \dfrac{{{y^2}}}{3} = 1$, which is in the general form of the equation of an ellipse. In the given case, the length of the major axis is $2a = 2 \times \sqrt {27} = 6\sqrt 3 $ and the length of the minor axis is $2b = 2 \times \sqrt 3 = 2\sqrt 3 $.

Now, let’s first talk about tangents of an ellipse. A tangent is a line that has one point common with the ellipse or a line that touches an ellipse at only one point. The parametric form of the equation of tangent of an ellipse is $\dfrac{{x\cos \theta }}{a} + \dfrac{{y\sin \theta }}{b} = 1$ at the point $\left( {a\cos \theta ,b\sin \theta } \right)$.
 $ \Rightarrow \dfrac{{x\cos \theta }}{{3\sqrt 3 }} + \dfrac{{y\sin \theta }}{{\sqrt 3 }} = 1$ will be the equation of tangent for this ellipse, at the point $\left( {3\sqrt 3 \cos \theta ,\sqrt 3 \sin \theta } \right)$

Now, we have to find point A and B, where this tangent will meet with coordinate axes.
$ \Rightarrow $ At $x = 0$, the equation will give us the point at which tangent meets y-axis
Therefore, when we put $x = 0$: $\dfrac{{x\cos \theta }}{{3\sqrt 3 }} + \dfrac{{y\sin \theta }}{{\sqrt 3 }} = 1 \Rightarrow 0 + \dfrac{{y\sin \theta }}{{\sqrt 3 }} = 1 \Rightarrow y = \dfrac{{\sqrt 3 }}{{\sin \theta }}$
Hence, coordinates of point A will be $\left( {0,\dfrac{{\sqrt 3 }}{{\sin \theta }}} \right)$
Similarly, at $y = 0$, the equation will give us the point at which tangent meets the x-axis
When we put $y = 0$ in equation, we get: $\dfrac{{x\cos \theta }}{{3\sqrt 3 }} + \dfrac{{y\sin \theta }}{{\sqrt 3 }} = 1 \Rightarrow \dfrac{{x\cos \theta }}{{3\sqrt 3 }} + 0 = 1 \Rightarrow x = \dfrac{{3\sqrt 3 }}{{\cos \theta }}$
Thus, coordinates of point B will be $\left( {\dfrac{{3\sqrt 3 }}{{\cos \theta }},0} \right)$

So, now we got a right-angled triangle $ \Delta AOB$, with altitude $\dfrac{{\sqrt 3 }}{{\sin \theta }}$ units and base $\dfrac{{3\sqrt 3 }}{{\cos \theta }}$ units.
Area of triangle$ = \dfrac{1}{2} \times Base \times Altitude$
Hence, the area of the triangle $\Delta AOB = \dfrac{1}{2} \times \dfrac{{3\sqrt 3 }}{{\cos \theta }} \times \dfrac{{\sqrt 3 }}{{\sin \theta }} = \dfrac{{3 \times 3}}{{2\cos \theta \sin \theta }}$
We can surely use the double-angle identity here, i.e.$\sin 2\theta = 2\sin \theta \cos \theta $, which will give us:
Area of triangle $\Delta AOB = \dfrac{{3 \times 3}}{{2\cos \theta \sin \theta }} = \dfrac{9}{{\sin 2\theta }}$

But according to the question, we need to find the minimum possible area of the triangle $\Delta OAB$. Since we already have an expression for the value of the area of the triangle, we should rather try to find the minimum value of$\dfrac{9}{{\sin 2\theta }}$, which will eventually give us the required answer.
$ \Rightarrow $ The minimum value of $\dfrac{9}{{\sin 2\theta }}$ will be attained when the value of the denominator will be at its maximum, i.e. when $\sin 2\theta $ will be maximum.
$ \Rightarrow $ As we know, $\sin 2\theta \in \left( { - 1,1} \right)$and the maximum value for a sine function is $1$.
Therefore, at $\sin 2\theta = 1$ , the area of the triangle $\Delta OAB$ will be $\dfrac{9}{{\sin 2\theta }} = \dfrac{9}{1} = 9sq.units$

So, the correct answer is “Option B”.

Note:Try to make a rough figure with the information given. This will help you understand and visualize the problem better. The sine function is the ratio of perpendicular and hypotenuse, which is the biggest side of a triangle. So, that is why the value of a sine function can never be greater than $1$. An alternative approach to this problem is the use of the equation of tangent in slope form or point form. But figuring out the maximum or minimum value for a trigonometric expression will be easier since they have fixed ranges.