
If
A.
B.
C.
D.
Answer
513k+ views
Hint: We find the expression for slope of the tangent at any point by differentiating the given curve and then the slope of the normal using perpendicular condition. We find the slopes of the intersecting normals as
Complete step by step answer:
We know from differential calculus that the slope of any curve at any point is given by the differentiation with respect to the independent variable. We also know that the slope of the curve at any point is the slope of the tangent at that point.
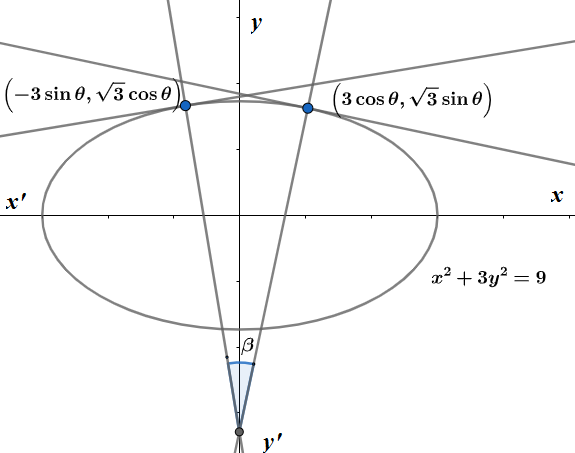
We are given equation of the ellipse as
Let us differentiate equation of above ellipse with respect to
We know that the product of slopes of two perpendicular line is
Since we are given there are two normals with points of contact points
We are given that the angle between the normals is
Since tangent and co-tangent are reciprocal to each other we have;
We convert the tangent and co-tangents into sine and cosine to have;
We use the Pythagorean trigonometric identity
Hence the correct option is C.
Note:
We note that since we are given
Complete step by step answer:
We know from differential calculus that the slope of any curve at any point is given by the differentiation with respect to the independent variable. We also know that the slope of the curve at any point is the slope of the tangent at that point.
We are given equation of the ellipse as
Let us differentiate equation of above ellipse with respect to
We know that the product of slopes of two perpendicular line is
Since we are given there are two normals with points of contact points
We are given that the angle between the normals is
Since tangent and co-tangent are reciprocal to each other we have;
We convert the tangent and co-tangents into sine and cosine to have;
We use the Pythagorean trigonometric identity
Hence the correct option is C.

Note:
We note that since we are given
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
Why is there a time difference of about 5 hours between class 10 social science CBSE

Five things I will do to build a great India class 10 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Select the word that is correctly spelled a Twelveth class 10 english CBSE

10 examples of evaporation in daily life with explanations




