
If a spherical steel ball is melted to make 8 new identical balls then the radius of the new ball is how many times the radius of the original ball?
Answer
518.1k+ views
Hint: In this particular question use the concept that the volume of the spherical steel ball is equal to the volume of the 8 new identical balls and the volume of the sphere is
Complete step-by-step answer:
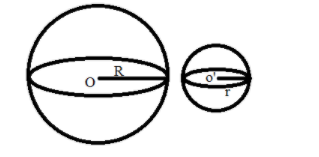
Consider a spherical steel ball with center O having radius R is shown in the above figure.
Now this spherical steel ball is melted into 8 new identical balls, one of the new steel balls is shown in the above figure having center O’, and radius r.
Now as we know that the volume of the sphere is
As we know that when we melted the spherical steel ball to make 8 new identical balls the volume remains constant.
So the volume of the big spherical ball = volume of the 8 new spherical balls.
Where R is the radius of the big spherical steel ball and r is the radius of the new spherical steel balls.
Now simplify it we have,
Now take cube root on both sides we have,
So the radius of the new balls is half of the radius of the original spherical steel balls.
Note: Whenever we face such types of questions the key concept we have to remember is that always recall the when we melted something to make a new thing volume always remain constant and always recall the formula of the volume of the sphere which is stated above so simply equate the volume of the original spherical steel ball with the volume of the 8 new identical balls as above and simplify we will get the required radius of the new ball in terms of original ball.
Complete step-by-step answer:

Consider a spherical steel ball with center O having radius R is shown in the above figure.
Now this spherical steel ball is melted into 8 new identical balls, one of the new steel balls is shown in the above figure having center O’, and radius r.
Now as we know that the volume of the sphere is
As we know that when we melted the spherical steel ball to make 8 new identical balls the volume remains constant.
So the volume of the big spherical ball = volume of the 8 new spherical balls.
Where R is the radius of the big spherical steel ball and r is the radius of the new spherical steel balls.
Now simplify it we have,
Now take cube root on both sides we have,
So the radius of the new balls is half of the radius of the original spherical steel balls.
Note: Whenever we face such types of questions the key concept we have to remember is that always recall the when we melted something to make a new thing volume always remain constant and always recall the formula of the volume of the sphere which is stated above so simply equate the volume of the original spherical steel ball with the volume of the 8 new identical balls as above and simplify we will get the required radius of the new ball in terms of original ball.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE




