
If a line intercepted between the coordinate axes is trisected at a point
A.
B.
C.
D.
Answer
512.4k+ views
1 likes
Hint:Here we assume the x and y intercepts of the line and write the equation of line in intercept form. Then using the concept of trisection at point A we divide the line into two segments with the help of ratio. Using the section formula we write the coordinates of the point dividing the line into ratio and equate the coordinates to given coordinates to obtain the value of h and k.
Formula used:
a) General equation of a line having x intercept as h and y intercept as k is
b)Section formula states that if a point
Complete step-by-step answer:
First we draw a diagram of a line having intercept h on x-axis and intercept k on y-axis. Intercept means the point where the line cuts that axis. Here x intercept is h so the point where the line meets the x-axis is
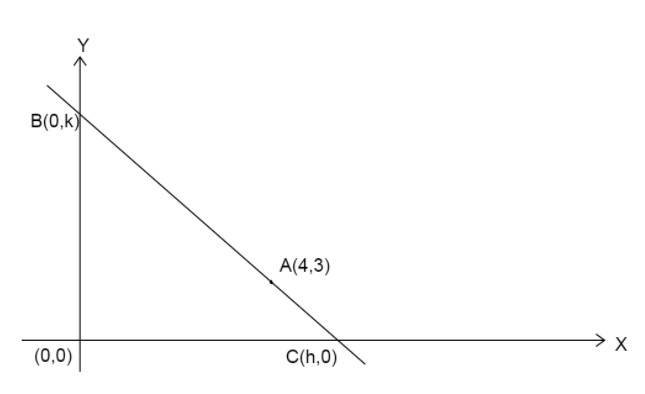
We have point
Let us assume equation of line be
We are given that the point
So, the point
Now we know from section formula that the point which divides the line joining two points
Here points are
Substitute the value of
Now we equate the coordinates of the point to coordinate of
Cross multiply the denominator of LHS to RHS of the equation
Divide both sided by 2
Now,
Cross multiply the denominator of LHS to RHS of the equation
So, the value of
Substitute in equation (1) to obtain equation of the line
Take LCM
Cross multiply the denominator of LHS to RHS of the equation
We get,
So, the correct answer is “Option B”.
Note:Students are likely to make mistake in substituting the values for a,b,c,d in the section formula as we choose the points according to the ratio, here ratio is
Formula used:
a) General equation of a line having x intercept as h and y intercept as k is
b)Section formula states that if a point
Complete step-by-step answer:
First we draw a diagram of a line having intercept h on x-axis and intercept k on y-axis. Intercept means the point where the line cuts that axis. Here x intercept is h so the point where the line meets the x-axis is

We have point
Let us assume equation of line be
We are given that the point
So, the point
Now we know from section formula that the point which divides the line joining two points
Here points are
Substitute the value of
Now we equate the coordinates of the point to coordinate of
Cross multiply the denominator of LHS to RHS of the equation
Divide both sided by 2
Now,
Cross multiply the denominator of LHS to RHS of the equation
So, the value of
Substitute in equation (1) to obtain equation of the line
Take LCM
Cross multiply the denominator of LHS to RHS of the equation
We get,
So, the correct answer is “Option B”.
Note:Students are likely to make mistake in substituting the values for a,b,c,d in the section formula as we choose the points according to the ratio, here ratio is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




