
If a couple has three daughters, what are the chances that the fourth child will be a son?
A.
B.
C.
D.
Answer
511.8k+ views
Hint: The gender of an individual is decided by the genes the offspring inherits from the parents. The father can donate either a Y chromosome or an X chromosome. The mother can only donate an X chromosome. If the offspring receives two X chromosomes, they're going to be female. If the offspring inherits an X and a Y chromosome, they're going to be male.
Complete answer: There are two sex chromosomes X and Y. Humans are diploid having two sex chromosomes, in females a pair of the X chromosome is found while in males an X and Y chromosome is present. There is
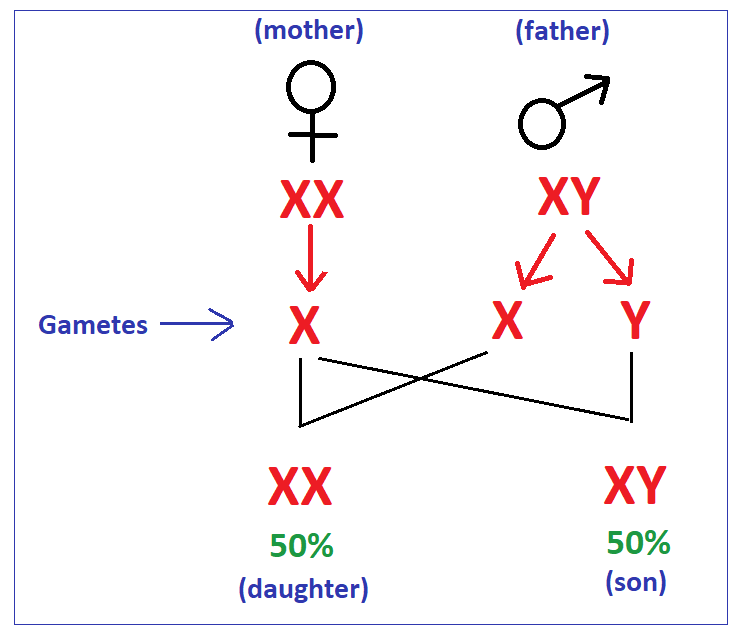
So, the correct answer is option C, i.e.,
Note: It is important to note that when the calculations are done with precision then, it is found that for the first child born the probability is
Complete answer: There are two sex chromosomes X and Y. Humans are diploid having two sex chromosomes, in females a pair of the X chromosome is found while in males an X and Y chromosome is present. There is

So, the correct answer is option C, i.e.,
Note: It is important to note that when the calculations are done with precision then, it is found that for the first child born the probability is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

What is transplantation in agriculture class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Hydrological cycle is controlled by A Grasslands B class 12 biology CBSE

Who discovered the cell and how class 12 biology CBSE




