
How do you graph
Answer
480.3k+ views
Hint: In this question, first we have to graph the given function which is in polar coordinate form in terms of
Complete step-by-step answer:
Polar coordinate pairs can have positive angles or negative angles for values of theta. In addition, they can have positive and negative radii. When graphing polar coordinates, though, the radius can be negative, which means that you move in the opposite direction of the angle from the pole.
Now given function is
Take different values for
So first take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now further simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now tabulating the values we get,
Now plotting the graphs we get,
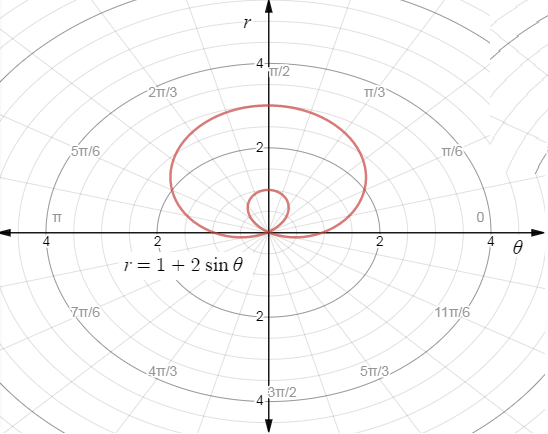
The plane appears this way because the polar coordinates are a given radius and a given angle in standard position from the pole. Each circle represents one radius unit, and each line represents the special angles from the unit circle.
Note:
Polar coordinates are based on angles, unlike Cartesian coordinates, polar coordinates have many different ordered pairs. Because infinitely many values of theta have the same angle in standard position, an infinite number of coordinate pairs describe the same point. Also, a positive and a negative coterminal angle can describe the same point for the same radius, and because the radius can be either positive or negative, you can express the point with polar coordinates in many ways.
Complete step-by-step answer:
Polar coordinate pairs can have positive angles or negative angles for values of theta. In addition, they can have positive and negative radii. When graphing polar coordinates, though, the radius can be negative, which means that you move in the opposite direction of the angle from the pole.
Now given function is
Take different values for
So first take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now further simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
Now take
Now substitute the value in the given function
Now simplifying we get,
Now again simplifying we get,
Now adding to simplify further we get,
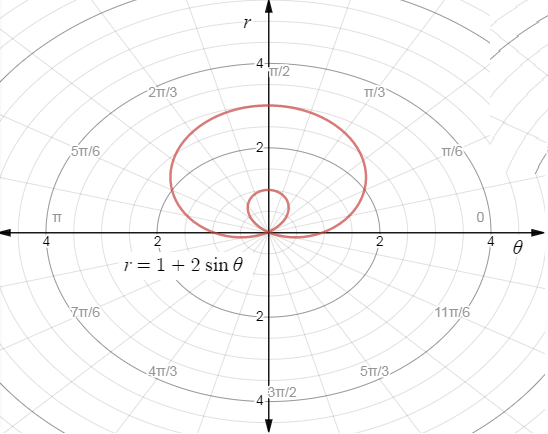
Now tabulating the values we get,
| 0 | 1 |
| 2.414 | |
| 3 | |
| 2.414 | |
| 1 | |
| -0.414 | |
| -1 | |
| -0.414 | |
| 1 |
Now plotting the graphs we get,

The plane appears this way because the polar coordinates are a given radius and a given angle in standard position from the pole. Each circle represents one radius unit, and each line represents the special angles from the unit circle.

Note:
Polar coordinates are based on angles, unlike Cartesian coordinates, polar coordinates have many different ordered pairs. Because infinitely many values of theta have the same angle in standard position, an infinite number of coordinate pairs describe the same point. Also, a positive and a negative coterminal angle can describe the same point for the same radius, and because the radius can be either positive or negative, you can express the point with polar coordinates in many ways.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




