
How do you graph
Answer
467.4k+ views
Hint:
We have to plot two inequalities on the graph. The plotting of an inequation is an area where all the points satisfy the inequation. We have found such an area where all the points satisfy the given two conditions or constraints. For plotting an inequation we first plot the equation that we get by just replacing the inequality sign with ‘=’ sign. And then we choose the area satisfied by the inequation.
Complete step by step solution:
We are given two inequalities
But for the ease of explanation here we will plot the graphs differently and then present the combined result.
First we will plot
For plotting the straight line we need two points which we can get by putting two values of
For
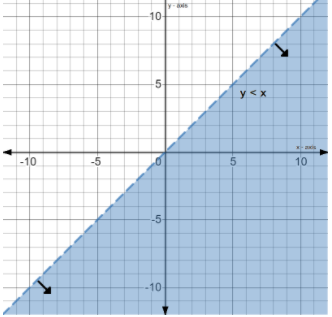
Fig. 1 - Graph of
Now we plot
Here the value of
The graph is now divided into two areas corresponding to
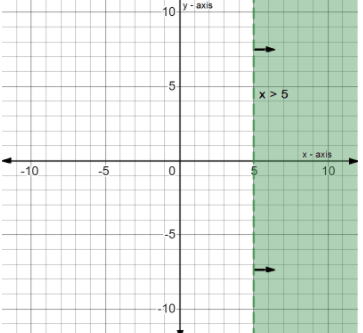
Fig. 2 - Graph of
We have to plot both the above graphs in the same graph to get the desired result. The resulting graph is shown in Fig. 3.
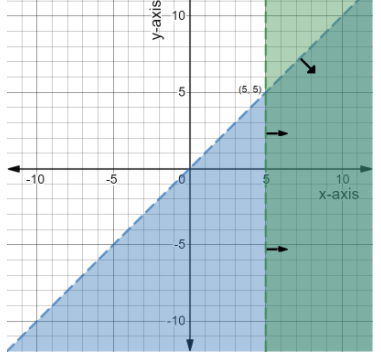
Fig. 3 - Graph of
The resulting area is the darkened region in Fig. 3, also indicated by the arrows. All the points in this region will satisfy both the conditions
Note:
The line of the graphs
We have to plot two inequalities on the graph. The plotting of an inequation is an area where all the points satisfy the inequation. We have found such an area where all the points satisfy the given two conditions or constraints. For plotting an inequation we first plot the equation that we get by just replacing the inequality sign with ‘=’ sign. And then we choose the area satisfied by the inequation.
Complete step by step solution:
We are given two inequalities
But for the ease of explanation here we will plot the graphs differently and then present the combined result.
First we will plot
For plotting the straight line we need two points which we can get by putting two values of
For

Fig. 1 - Graph of
Now we plot
Here the value of
The graph is now divided into two areas corresponding to

Fig. 2 - Graph of
We have to plot both the above graphs in the same graph to get the desired result. The resulting graph is shown in Fig. 3.

Fig. 3 - Graph of
The resulting area is the darkened region in Fig. 3, also indicated by the arrows. All the points in this region will satisfy both the conditions
Note:
The line of the graphs
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
EMI starts from ₹3,775 per month
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




