
How do you graph and list the amplitude, period, phase shift for
Answer
452.7k+ views
Hint: First, using the suitable trigonometric identities, simplify the given equation and try to get a simplest form of the equation so that we can understand it better. Then find the maximum value of the function.
Complete step by step solution:
The given equation is
This is a trigonometric equation. All trigonometric functions are periodic. This means that the function repeats itself after a regular interval on the Cartesian plane.
The trigonometric function
This helps in graphing the curve of a trigonometric function. We can graph the function for an interval of
Let us simplify equation
Then,
Now, we shall use the identity
So, we get,
This means that the graph of equation
So, we get the graph of
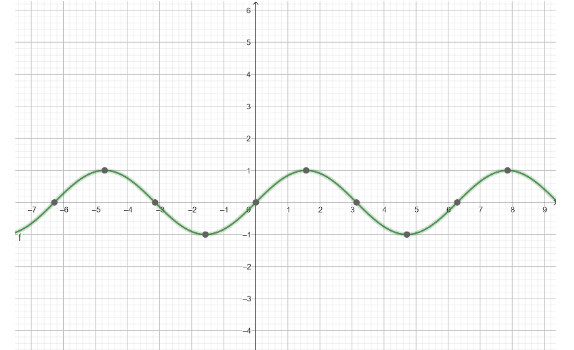
So, we now know that
Hence, the maximum value of the function
Therefore, the amplitude of the function
Period of the function
Phase shift of the graph is zero.
Note: If we have an equation
Here, in this case,
This means that amplitude of the function is
Complete step by step solution:
The given equation is
This is a trigonometric equation. All trigonometric functions are periodic. This means that the function repeats itself after a regular interval on the Cartesian plane.
The trigonometric function
This helps in graphing the curve of a trigonometric function. We can graph the function for an interval of
Let us simplify equation
Then,
Now, we shall use the identity
So, we get,
This means that the graph of equation
So, we get the graph of

So, we now know that
Hence, the maximum value of the function
Therefore, the amplitude of the function
Period of the function
Phase shift of the graph is zero.
Note: If we have an equation
Here, in this case,
This means that amplitude of the function is
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

The non protein part of an enzyme is a A Prosthetic class 11 biology CBSE

Which of the following blood vessels in the circulatory class 11 biology CBSE

What is a zygomorphic flower Give example class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

The deoxygenated blood from the hind limbs of the frog class 11 biology CBSE




