Question

From the top of a tower 100 m high, a man observes two cars on the opposite sides of the tower and in the same straight line with its base, with angle of depression ${30^0}$ and ${45^0}$ respectively. Find the distance between the cars. (Take $\sqrt 3 = 1.732$)

Answer 1

Hint: Here, we will proceed by drawing the appropriate figure according to the problem statement and then we will use the concept that alternative angles are equal. After that we will apply the formula $\tan \theta = \dfrac{{{\text{Perpendicular}}}}{{{\text{Base}}}}$ in order to find the lengths CB and BD.

Complete step-by-step answer:

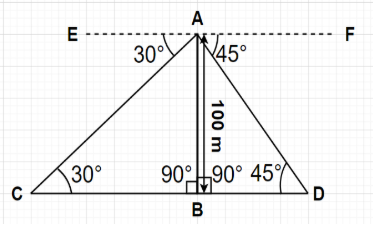
Let us suppose AB is a tower of height 100 m where a man is observing from point A i.e., the top of the tower as shown in the figure. Let C and D are the positions of the two cars on the opposite sides of the tower AB and are in the same straight line with the base (i.e., B) of the tower.
Given, the angles of depression of the two cars C and D when observed by a man from the top of the tower i.e., point A are $\angle {\text{EAC}} = {30^0}$ and $\angle {\text{FAD}} = {45^0}$ respectively.
Clearly, from the figure the two pairs of alternate angles are $\angle {\text{EAC}}$ and $\angle {\text{ACB}}$, $\angle {\text{FAD}}$ and $\angle {\text{ADB}}$. Since, the alternative angles are equal in measure.
So, $\angle {\text{EAC}} = \angle {\text{ACB}} = {30^0}$ and $\angle {\text{FAD}} = \angle {\text{ADB}} = {45^0}$
As we know that in any right angled triangle, $\tan \theta = \dfrac{{{\text{Perpendicular}}}}{{{\text{Base}}}}$
In right angled triangle ABC,
$\tan {30^0} = \dfrac{{{\text{AB}}}}{{{\text{CB}}}}{\text{ }} \to {\text{(1)}}$
As, AB=100 m and according to the general trigonometric table,$\tan {30^0} = \dfrac{1}{{\sqrt 3 }}$
By substituting the above values in equation (1), we get
$
   \Rightarrow \dfrac{1}{{\sqrt 3 }} = \dfrac{{{\text{100}}}}{{{\text{CB}}}} \\
   \Rightarrow {\text{CB}} = {\text{100}}\sqrt 3 {\text{ m}} \\
 $
In right angled triangle ABD,
$\tan {45^0} = \dfrac{{{\text{AB}}}}{{{\text{BD}}}}{\text{ }} \to {\text{(2)}}$
As, AB=100 m and according to the general trigonometric table,$\tan {45^0} = 1$
By substituting the above values in equation (2), we get
$
   \Rightarrow 1 = \dfrac{{{\text{100}}}}{{{\text{BD}}}} \\
   \Rightarrow {\text{BD}} = {\text{100 m}} \\
 $
As, CD=CB+BD=${\text{100}}\sqrt 3 $+100=${\text{100}}\left( {\sqrt 3 + 1} \right)$
Put $\sqrt 3 = 1.732$ in the above equation, we get
CD=100(1.732+1)=100(2.732)=273.2 m
Therefore, the distance between the cars C and D is CD which is equal to 273.2 m.

Note: In any right angled triangle, the side opposite to the right angle is the hypotenuse, the side opposite to the considered angle is the perpendicular and the remaining side is the base. So, in the right angled triangle ABC, AC, AB and CB are the hypotenuse, perpendicular and base respectively. Similarly, in the right angled triangle ABD, AD, AB and BD are the hypotenuse, perpendicular and base respectively.