Question

From a point in the interior of an equilateral triangle, perpendiculars are drawn on the three sides and they are of lengths 10 cm, 12 cm, and 18 cm. Find the area of the triangle.

Answer 1

Hint: In these types of questions use the basic formulas of the triangle and also use the perpendicular to form triangles and apply the addition operation to find the area of the equilateral triangle.
Complete step-by-step answer:

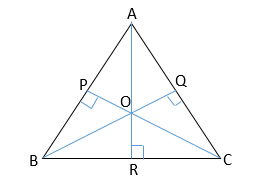
Let the side AB, BC, AC of the equilateral triangle be equal to each other by the property of the equilateral triangle.
Let x be the side of the equilateral triangle.
Therefore AB=AC=BC=x
Now area of equilateral triangle= $\dfrac{{\sqrt 3 }}{4}{(\text{side})^2}$= $\dfrac{{\sqrt 3 }}{4}{(x)^2}$ ---(equation 1)
By the above diagram
Area of an equilateral triangle ABC = area of triangle AOC + area of triangle AOB + area of BOC
Area of AOC= $\dfrac{1}{2}\text{Base} \times {\text{Height}}$=$\dfrac{1}{2}x \times 10$=5x
Area of BOC= $\dfrac{1}{2}\text{Base} \times {\text{Height}}$=$\dfrac{1}{2}x \times 12$=6x
Area of AOB= $\dfrac{1}{2}\text{Base} \times {\text{Height}}$=$\dfrac{1}{2}x \times 18$=9x
Since Area of an equilateral triangle ABC = area of triangle AOC + area of triangle AOB + area of BOC
$\dfrac{{\sqrt 3 }}{4}{(x)^2}$= 5x+6x+9x
$\dfrac{{\sqrt 3 }}{4}{(x)^2}$=20x
$ \Rightarrow $ x = $\dfrac{{20 \times 4}}{{\sqrt 3 }}$=\[\dfrac{{80}}{{\sqrt 3 }}\]
Now putting the value of x in equation 1
Therefore Area of an equilateral triangle= $\dfrac{{\sqrt 3 }}{4}{(\dfrac{{80}}{{\sqrt 3 }})^2}$
$ \Rightarrow $ $\dfrac{{\sqrt 3 }}{4} \times \dfrac{{6400}}{3}$=$\dfrac{{1600}}{{\sqrt 3 }}$
So $\dfrac{{1600}}{{\sqrt 3 }}{cm^2}$ is the area of the equilateral triangle.
Note: In these types of questions use the basic formula of the equilateral triangle to find the area. Then take a variable x as a side of the equilateral triangle and since the sides of the equilateral triangle are equal therefore every side will be equal to x. Now by the perpendicular drawn in the equilateral triangle, three triangles are formed using the area of the three triangles to find the area of the equilateral triangle Since the Area of an equilateral triangle ABC = area of triangle AOC + area of triangle AOB + area of BOC.