
Formula to find area of sector is
(a)
(b)
(c)
(d)
Answer
514.2k+ views
Hint: Here we will make use of area of a circle and some basic properties of circle geometry. Use the fact that ratio of area of a sector to the area of circle will be same as the ratio of central angle of sector to
Complete step by step solution:
A sector is a portion of a circle which is bounded by the radius and the adjoining arc. The semi-circle is also expressed as a sector of a circle, and it covers half a portion of the circle. Generally, a sector is categorized into two parts which are a major sector and a minor sector. Major sector has a larger central angle which is greater than
The following is the schematic diagram of the circle having radius r and angle
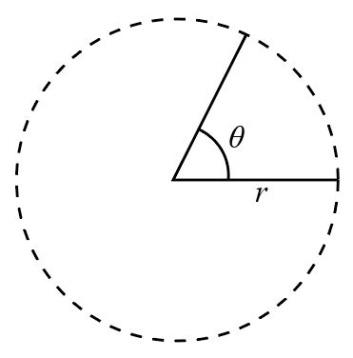
If central angle of sector is
Note: Here, the central angle should be taken in degrees always not in radians. If the central angle of the sector is given in radians then do not use 360 degrees in the denominator. First convert 360 degrees in radians. One other way, you can do is convert the given angle of the sector in degrees.
Complete step by step solution:
A sector is a portion of a circle which is bounded by the radius and the adjoining arc. The semi-circle is also expressed as a sector of a circle, and it covers half a portion of the circle. Generally, a sector is categorized into two parts which are a major sector and a minor sector. Major sector has a larger central angle which is greater than
The following is the schematic diagram of the circle having radius r and angle

If central angle of sector is
Note: Here, the central angle should be taken in degrees always not in radians. If the central angle of the sector is given in radians then do not use 360 degrees in the denominator. First convert 360 degrees in radians. One other way, you can do is convert the given angle of the sector in degrees.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What is the past tense of read class 10 english CBSE

In which type of rock coal is found A Igneous rock class 10 social science CBSE

Which one of the following is the deepest seaport of class 10 social science CBSE




