
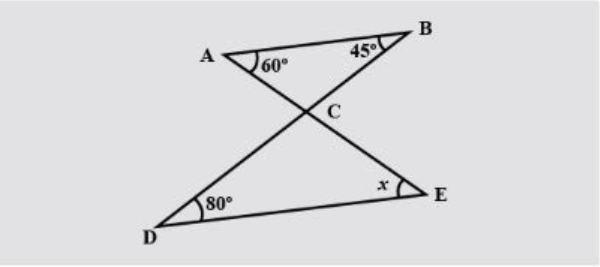
Find the value of x in each case:

Answer
539.7k+ views
1 likes
Hint: We know that the sum of internal angles of a triangle is
Complete step by step answer:
As per the given question, in
In the given figure
On substituting these values we get:
Now, lines AE and BD intersect each other at C. Therefore,
Therefore,
Again, for
And in the given figure ,
Note: When two lines intersect we have two pairs of vertically opposite angles and four pairs of adjacent angles. While the vertically opposite angles are equal, the pair of adjacent angles are supplementary, i.e. the sum of two angles is
Someone can be confused by looking at the picture that the lines AB and DE are parallel and then they can use the property of corresponding angles to write
Complete step by step answer:
As per the given question, in
In the given figure
On substituting these values we get:
Now, lines AE and BD intersect each other at C. Therefore,
Therefore,
Again, for
And in the given figure ,
Note: When two lines intersect we have two pairs of vertically opposite angles and four pairs of adjacent angles. While the vertically opposite angles are equal, the pair of adjacent angles are supplementary, i.e. the sum of two angles is
Someone can be confused by looking at the picture that the lines AB and DE are parallel and then they can use the property of corresponding angles to write
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




