
How do you find the third side of a right triangle if the base is x = 20m and height is y = 20m?
Answer
480.3k+ views
1 likes
Hint: Draw a rough diagram of a right-angle triangle ABC right angled at B. Now, assume the side BC as base and AB as height of the triangle. Apply the Pythagoras theorem given as: - base
Complete answer:
Here, we are provided with a right-angle triangle with the length of its base equal to 20m and length of its height equal to 20m. We have to determine the third side, that is hypotenuse of this triangle.
Now, let us draw a diagram of the given situation. So, we have,
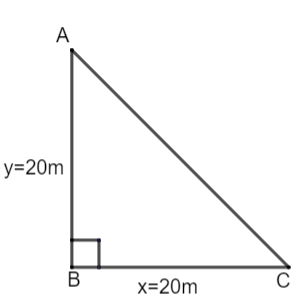
In the above figure we have assumed a right-angle triangle ABC right angled at B. We have considered BC as the base whose length is x = 20m and AB as the height or perpendicular whose length is y = 20m. AC is the hypotenuse whose length is unknown.
Now, we know that we can apply the Pythagoras theorem in a right-angle triangle to determine the unknown side if the length of the other two sides are given. So, we have,
In
AB = y = 20m = perpendicular (height) = p
BC = x = 20m = base = b
Applying Pythagoras theorem given as: -
Taking square root both sides, we get,
Hence, the length of the third side is
Note: One may note that besides Pythagoras theorem we can also apply the method of trigonometry to find the length of side AC. What we can do is we will first determine the angle C by using the formula: -
Complete answer:
Here, we are provided with a right-angle triangle with the length of its base equal to 20m and length of its height equal to 20m. We have to determine the third side, that is hypotenuse of this triangle.
Now, let us draw a diagram of the given situation. So, we have,

In the above figure we have assumed a right-angle triangle ABC right angled at B. We have considered BC as the base whose length is x = 20m and AB as the height or perpendicular whose length is y = 20m. AC is the hypotenuse whose length is unknown.
Now, we know that we can apply the Pythagoras theorem in a right-angle triangle to determine the unknown side if the length of the other two sides are given. So, we have,
In
AB = y = 20m = perpendicular (height) = p
BC = x = 20m = base = b
Applying Pythagoras theorem given as: -
Taking square root both sides, we get,
Hence, the length of the third side is
Note: One may note that besides Pythagoras theorem we can also apply the method of trigonometry to find the length of side AC. What we can do is we will first determine the angle C by using the formula: -
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




