
Find the maximum or minimum value of the quadratic expression
Answer
507k+ views
1 likes
Hint: We know that the minimum or maximum value of a quadratic expression
Complete step-by-step solution -
Before solving the question, we should whether a quadratic expression will have maximum value (or) minimum value.
For a quadratic expression
In the similar way, if
The given expression in this question is
Let us assume
By rewriting the quadratic expression,
Now we should compare
We know that if
From equation (1), it is clear that the value of a for
So, the quadratic expression
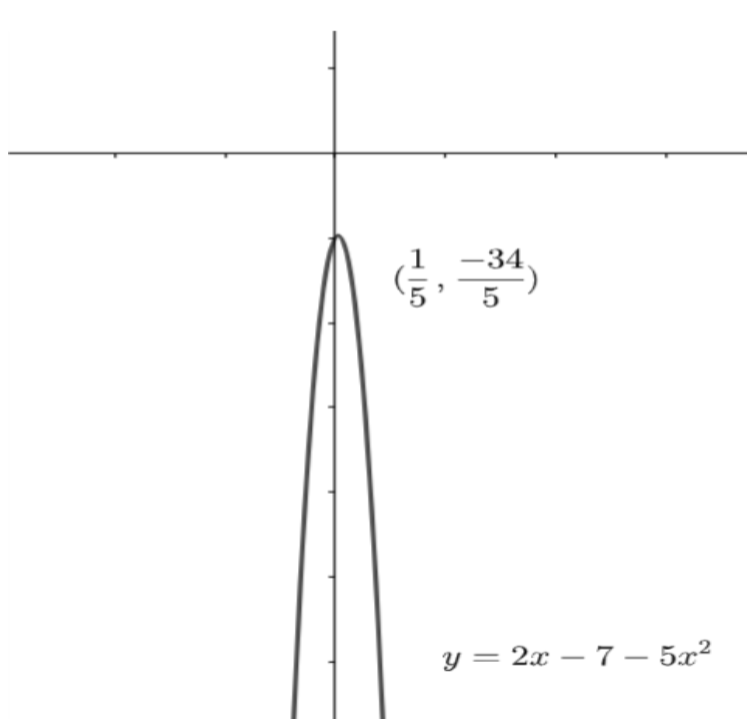
We know that the minimum value for a quadratic expression will obtain at
From equation (2) and equation (3), the maximum value of quadratic expression will obtain at
We know that the maximum value of quadratic expression is
So, the maximum value of quadratic expression is
Hence, the maximum value of
Note: There is an alternative method to solve this problem.
A function f(x) is said to have a maximum or minimum value at the value of x where
The value of x where f`(x)=0 is said to have a maximum value if
The value of x where f`(x)=0 is said to have a minimum value if
Let us assume
We have to find the value of x where f`(x) is equal to 0.
At
As
So, substitute equation (2) in equation (1).
Hence, the maximum value of
Complete step-by-step solution -
Before solving the question, we should whether a quadratic expression will have maximum value (or) minimum value.
For a quadratic expression
In the similar way, if
The given expression in this question is
Let us assume
By rewriting the quadratic expression,
Now we should compare
We know that if
From equation (1), it is clear that the value of a for
So, the quadratic expression

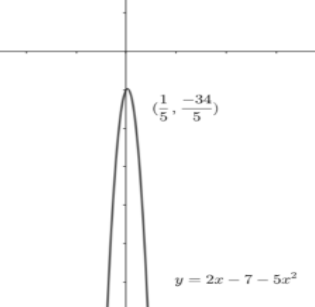
We know that the minimum value for a quadratic expression will obtain at
From equation (2) and equation (3), the maximum value of quadratic expression will obtain at
We know that the maximum value of quadratic expression is
So, the maximum value of quadratic expression is
Hence, the maximum value of
Note: There is an alternative method to solve this problem.
A function f(x) is said to have a maximum or minimum value at the value of x where
The value of x where f`(x)=0 is said to have a maximum value if
The value of x where f`(x)=0 is said to have a minimum value if
Let us assume
We have to find the value of x where f`(x) is equal to 0.
At
As
So, substitute equation (2) in equation (1).
Hence, the maximum value of

Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




