
Find the length of the medians of a triangle whose vertices are A (-1,3) , B(1,-1) and C(5,1).
Answer
511.5k+ views
Hint: In order to find the length of the medians we need to fint the midpoint of the sides of the triangle using the formula
Complete step-by-step answer:
We are given the vertices of the triangle to be A (-1,3) , B(1,-1) and C(5,1)
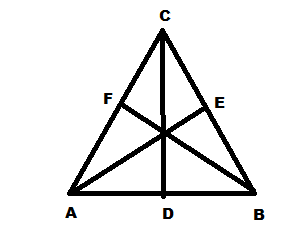
Medians are the lines joining the midpoint of a side of a triangle to the opposite vertice.
So we need to find the midpoint of the three sides first
We know the midpoint of the line joining the points
Midpoint of the line joining A(-1,3) and B(1,-1) , D=
Midpoint of the line joining B(1,-1) and C(5,1) , E=
Midpoint of the line joining A(-1,3) and C(5,1) , F=
Now we have the medians CD ,BF and AE
To find their length we need to use the distance formula
That is the distance between two points
Therefore the length of medians is given by
Distance between C(5,1) and D(0,1)
Length of CD=
Distance between B(1,-1) and F(2,2)
Length of BF=
Length of AE=
Therefore the length of the medians are 5 units ,
Note: The point of co incidence of the medians is known as centroid.
The point of co incidence of the altitudes is known as orthocentre.
Complete step-by-step answer:
We are given the vertices of the triangle to be A (-1,3) , B(1,-1) and C(5,1)

Medians are the lines joining the midpoint of a side of a triangle to the opposite vertice.
So we need to find the midpoint of the three sides first
We know the midpoint of the line joining the points
Midpoint of the line joining A(-1,3) and B(1,-1) , D=
Midpoint of the line joining B(1,-1) and C(5,1) , E=
Midpoint of the line joining A(-1,3) and C(5,1) , F=
Now we have the medians CD ,BF and AE

To find their length we need to use the distance formula
That is the distance between two points
Therefore the length of medians is given by
Distance between C(5,1) and D(0,1)
Length of CD=
Distance between B(1,-1) and F(2,2)
Length of BF=
Length of AE=
Therefore the length of the medians are 5 units ,
Note: The point of co incidence of the medians is known as centroid.
The point of co incidence of the altitudes is known as orthocentre.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which of the following districts of Rajasthan borders class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

What is the full form of pH?

Define human made resources

India has states and Union Territories A 29 7 B 28 class 9 social science CBSE

What is the importance of natural resources? Why is it necessary to conserve them?




