Answer
408k+ views
- Hint: In this question we are asked to find the diameter with the given circumference. To find this first we need to find the radius of a circle by taking the formula of circumference of a circle $=2\pi r$. Then find diameter where $d=2r$.
Complete step-by-step solution -
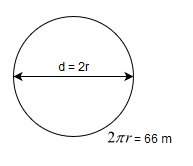
To find the diameter of a circle, let us first find the radius of a circle.
It is given that circumference of a circle = 66m.
We know that circumference of a circle $=2\pi r$ . So, equating it to 66 m, we get
$66=2\times \dfrac{22}{7}\times r$
Divide ‘2’ on both sides, we get –
$33=\dfrac{22}{7}\times r$
Multiply both sides by ‘7’ we get –
$231=22r$
Divide both sides by ‘22’ we get –
$\dfrac{231}{22}=r$
$r=10.5$
Now, let us find the diameter, where \[d=2r\] .
Here, we will put the value of $r=10.5$
$\begin{align}
& d=2\times 10.5 \\
& d=21 \\
\end{align}$
Hence, the diameter of the circle is 21 m.
Note: Circumference of Circle: The distance around the circular region is called its circumference. The ratio of circumference of any circle to its diameter is constant. This constant is denoted by $\pi $ and is read as pie. $\dfrac{\text{circumference}}{diameter}=\pi $ or Circumference of a circle $=2\pi r$ , where ‘r’ denoted as the radius of the circle. So, using this relation also, we can directly get the diameter.
Complete step-by-step solution -

To find the diameter of a circle, let us first find the radius of a circle.
It is given that circumference of a circle = 66m.
We know that circumference of a circle $=2\pi r$ . So, equating it to 66 m, we get
$66=2\times \dfrac{22}{7}\times r$
Divide ‘2’ on both sides, we get –
$33=\dfrac{22}{7}\times r$
Multiply both sides by ‘7’ we get –
$231=22r$
Divide both sides by ‘22’ we get –
$\dfrac{231}{22}=r$
$r=10.5$
Now, let us find the diameter, where \[d=2r\] .
Here, we will put the value of $r=10.5$
$\begin{align}
& d=2\times 10.5 \\
& d=21 \\
\end{align}$
Hence, the diameter of the circle is 21 m.
Note: Circumference of Circle: The distance around the circular region is called its circumference. The ratio of circumference of any circle to its diameter is constant. This constant is denoted by $\pi $ and is read as pie. $\dfrac{\text{circumference}}{diameter}=\pi $ or Circumference of a circle $=2\pi r$ , where ‘r’ denoted as the radius of the circle. So, using this relation also, we can directly get the diameter.
Recently Updated Pages
Three beakers labelled as A B and C each containing 25 mL of water were taken A small amount of NaOH anhydrous CuSO4 and NaCl were added to the beakers A B and C respectively It was observed that there was an increase in the temperature of the solutions contained in beakers A and B whereas in case of beaker C the temperature of the solution falls Which one of the following statements isarecorrect i In beakers A and B exothermic process has occurred ii In beakers A and B endothermic process has occurred iii In beaker C exothermic process has occurred iv In beaker C endothermic process has occurred

The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Trending doubts
Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Change the following sentences into negative and interrogative class 10 english CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How many crores make 10 million class 7 maths CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE

What organs are located on the left side of your body class 11 biology CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE



